إجابات أتحقق من فهمي
نظريتا الباقي والعامل
 صفحة (54):
صفحة (54):
أستعمل طريقة الجدول؛ لأجد ناتج كلّ ممّا يأتي:
a) (x3 + 6x2 – 9x – 14) (x + 1)
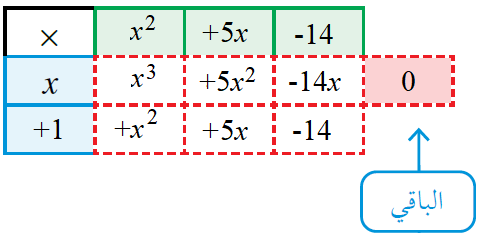
ناتج القسمة هو x2 + 5x - 14 ، والباقي 0
b) (2x3 - x2 + 3) (x - 3)
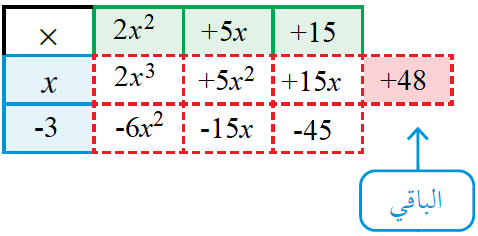
ناتج القسمة هو x2 + 5x + 152 ، والباقي 48
 صفحة (57):
صفحة (57):
أستعمل نظرية الباقي؛ لأجد باقي قسمة P(x) على h(x) في كلّ ممّا يأتي:
a)
b)
c)
 صفحة (58):
صفحة (58):
إذا كان P(x) = x3 – 2x 2 – 13 x - 10
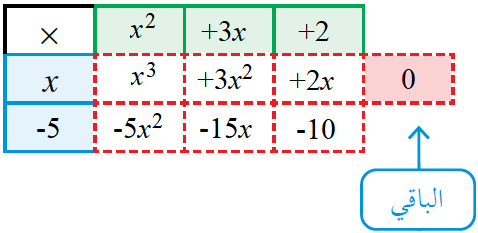
لتحليل P(x) أقسم P(x) على (x - 5)b)
P(x) = (x – 5) (x2 + 3x + 2)
= (x – 5) (x + 2) (x + 1)
 صفحة (61):
صفحة (61):
أجد أصفار كثيرات الحدود الآتية جميعها:
a) P(x) = 5x3 – x2 – 5x + 1
عوامل الحد الثابت هي: ، وعوامل المعامل الرئيس هي
، و
، الأصفار المحتملة للاقتران هي:
،
بالتعويض نجد أن P(1) = 5 – 1 – 5 + 1 = 0
إذن: (x-1) هو أحد عوامل P(x)
أجد العوامل الأخرى بالقسمة وتحليل الناتج إن أمكن.
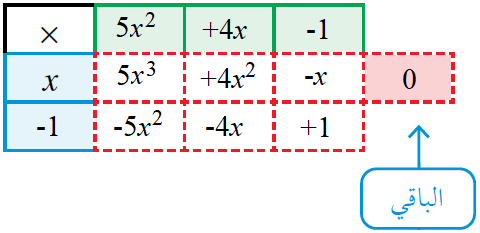
P(x) = (x-1) (5x2 + 4x – 1)
= (x-1) (5x-1) (x+1)
إذن، أصفار P(x) هي: ، -1 ، 1
b) Q(x) = x4 + 6x3 + 7x2 – 6x - 8
معامل الحد الرئيس يساوي 1 ، فالأصفار المحتملة هي عوامل الحد الثابت 8 ، وهي: ،
،
،
بالتعويض نجد أنّ: Q(1) = 1 + 6 + 7 – 6 – 8 = 0
إذن: (x-1) هو أحد عوامل Q(x)
أجد العوامل الأخرى بالقسمة وتحليل الناتج إن أمكن.
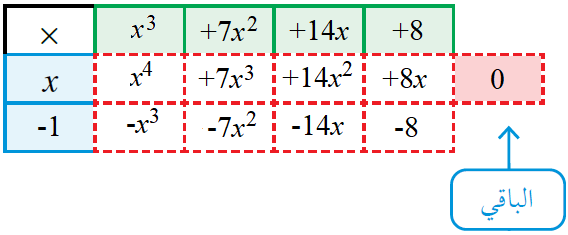
Q(x) = (x-1) (x3 + 7x2 + 14x + 8)
وبتعويض x = -1 في العامل التكعيبي نجد أن الناتج 0 ،
نقسم x3 + 7x2 + 14x + 8 على (x+1).

فنجد أنّ:
Q(x) = (x-1) (x+1) (x2 + 6x + 8)
= (x-1) (x+1) (x+2) (x+4)
إذن أصفار Q(x) هي: 1- , 1 , -2 , -4
 صفحة (63):
صفحة (63):
أحل كلّ معادلة ممّا يأتي:
a) x3 - x2 – 9x + 9 = 0
x2(x -1) – 9(x -1) = 0
(x -1) (x 2-9) = 0
(x -1) (x -3) (x +3) = 0
x = 1 , x = 3 , x = -3
إذن، حلول هذه المعادلة هي: x = 1 , x = 3 , x = -3
b) x3 + 3x2 – 4 = 0
حلول هذه المعادلة هي: x = 1 , x = -2 ، ويمكن حلها بتحليل الطرف الأيسر إلى عوامل بطريقة مشابهة لحل الفقرة (a)، ويمكن حلها بطريقة المثال (5).
 صفحة (64):
صفحة (64):
يزيد ارتفاع أسطوانة 5 cm على طول نصف قطر قاعدتها. إذا كان حجم الاسطوانة 72π cm3 ؛ فما طول نصف قطر قاعدتها وارتفاعها؟
نصف قطر قاعدة الاسطوانة 3 cm ، وارتفاعها 8 cm
إعداد : شبكة منهاجي التعليمية
02 / 10 / 2022
النقاشات