تطبيقات القيم القصوى

أتحقق من فهمي

أتحقق من فهمي

تطبيقات القيم القصوى

إيجاد أكبر حجم ممكن

أتحقق من فهمي صفحة 121

ترغب شركة في تصميم صندوق مفتوح من الأعلى، وقاعدته مربعة الشكل، ومساحة سطحه الكلية 1080 cm² كما في الشكل المجاور. أجد أبعاد الصندوق ليكون حجمه أكبر ما يمكن.

ليكن حجم الصندوق V ومساحة سطحه الكلية A

$\begin{aligned} A = 4 x h + x^{2} = 1080 \to h = \frac{1080 - x^{2}}{4 x} \\ V = x^{2} h \\ V (x) = x^{2} (\frac{1080 - x^{2}}{4 x}) = \frac{1}{4} (1080 x - x^{3}), 0 \leq x \leq \sqrt{1080} \\ V^{'} (x) = \frac{1}{4} (1080 - 3 x^{2}) \\ V^{'} (x) = 0 \to x = \sqrt{360} \end{aligned}$

القيمة الحرجة هي: $\sqrt{360}$

أجد حجم الصندوق عند القيمة الحرجة وعند طرفي المجال.

$\begin{aligned} V (0) = 0 \\ V (\sqrt{360}) = \frac{1}{4} (1080 \sqrt{360} - 360 \sqrt{360}) = 180 \sqrt{360} = 1080 \sqrt{10} \\ V (\sqrt{1080}) = 0 \end{aligned}$

إذن يكون الحجم أكبر ما يمكن عندما $x = 6 \sqrt{10} cm$ وعندها يكون الارتفاع $h = 3 \sqrt{10} cm$

إيجاد أقل طول ممكن

أتحقق من فهمي صفحة 124

خطط مزارع لتسييج حضيرة مستطيلة الشكل قرب نهر كما في الشكل المجاور، وحدّد مساحة الحظيرة بـ 245000 m² ؛ لتوفير كمية عشب كافية لأغنامه.

أجد أبعاد الحظيرة التي تجعل طول السياج أقل ما يمكن، علماً بأنّ الجزء المقابل للنهر لا يحتاج إلى تسييج.

ليكن طول السياج L ومساحة الحظيرة A

$\begin{aligned} A = x y = 245000 \to y = \frac{245000}{x} \\ L = x + 2 y \\ L (x) = x + \frac{490000}{x}, x > 0 \\ L^{'} (x) = 1 - \frac{490000}{x^{2}} \\ L^{'} (x) = 0 \to x = 700 \end{aligned}$

قيمة x الحرجة هي: 700

$L^{''} (x) = \frac{980000}{x^{3}} \to L^{''} (700) = \frac{980000}{(700)^{3}} > 0$

إذن يكون طول السياج أقل ما يمكن عندما x = 700 m و $y = \frac{245000}{700} = 350 m$

إيجاد أقرب مسافة

أتحقق من فهمي صفحة 126

$قطار$ انطلق قطار من إحدى المحطات الساعة 10:00 a.m. ، وتحرك في اتجاه الجنوب بسرعة 60 km/h ، حيث المحطة التالية. وفي الوقت نفسه، انطلق قطار آخر نحو الغرب بسرعة 45 km/h ، ثم وصل إلى محطة الأول الساعة 11:00 a.m. . في أي ساعة يكون القطاران أقرب ما يمكن إلى بعضهما؟

نفرض x بُعد القطار الأول عن المحطة، y بُعد القطار الثاني عن المحطة، ونفرض D البعد بين القطارين.

القطار الثاني استغرق ساعة واحدة للوصول إلى المحطة، إذن فقد انطلق من نقطة تبعد 24 كيلومتراً عنها.

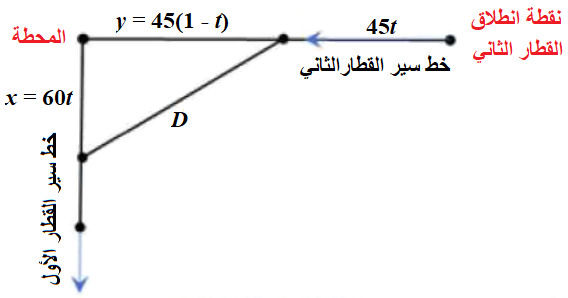
بعد t ساعة من انطلاقهما يكون: $x = 60 t$ ، ويكون $y = 45 - 45 t = 45 (1 - t)$

$\begin{aligned} D (t) = \sqrt{(60 t)^{2} + (45 (1 - t))^{2}} = \sqrt{3600 t^{2} + 2025 (1 - t)^{2}}, 0 \leq t \leq 1 \\ D^{'} (t) = \frac{7200 t - 4050 (1 - t)}{2 \sqrt{3600 t^{2} + 2025 (1 - t)^{2}}} = \frac{11250 t - 4050}{2 \sqrt{3600 t^{2} + 2025 (1 - t)^{2}}} \\ D^{'} (t) = 0 \to t = \frac{4050}{11250} = \frac{9}{25} \end{aligned}$

القيمة الحرجة هي: $t = \frac{9}{25}$

أجد المسافة D عند القيمة الحرجة وعند طرفي المجال.

$\begin{aligned} D (0) = \sqrt{2025} = 45 \\ D (1) = \sqrt{3600} = 60 \\ D (\frac{9}{25}) = \sqrt{3600 {(\frac{9}{25})}^{2} + 2025 {(1 - \frac{9}{25})}^{2}} = 36 \end{aligned}$

إذن يكون القطاران أقرب ما يمكن إلى بعضهما عندما $t = \frac{9}{25} h$ ، أي بعد 21 دقيقة و 36 ثانية، وتكون الساعة حينئذ 10:21:36

تطبيقات اقتصادية

أتحقق من فهمي صفحة 128

يبيع متجر 200 شاشة تلفاز شهرياً بسعر JD 350 للشاشة الواحدة. وقد أشار مسح للسوق أعدّه خبير التسويق في المتجر إلى أن عدد الشاشات المبيعة شهرياً يزيد بمقدار 20 شاشة عند كل خصم مقداره JD 10 من سعر الشاشة الواحدة. أجد سعر بيع الشاشة الواحدة الذي يحقق للمتجر أعلى إيراد ممكن.

ليكن سعر بيع الشاشة الواحدة هو x دينار.

أي أن مقدار الخصم من سعر بيع الشاشة الواحدة هو 350 - x دينار، وبالتالي تحصل زيادة في عدد الشاشات المبيعة مقدارها $\frac{20}{10} (350 - x) = 700 - 2 x$ شاشة.

إذن عدد الشاشات المبيعة سيكون: 200 + 700 – 2x = 900 – 2x

الإيراد = عدد الشاشات المبيعة × سعر بيع الشاشة الواحدة بعد الخصم:

$\begin{aligned} R (x) = (900 - 2 x) x = 900 x - 2 x^{2} \\ R^{'} (x) = 900 - 4 x \\ R^{'} (x) = 0 \to x = 225 \end{aligned}$

توجد قيمة حرجة وحيدة هي: 225

$R^{''} (x) = - 4 \to R^{''} (225) = - 4 < 0$

نلاحظ أن اقتران الإيراد له قيمة عظمى عندما x = 225

إذن يحقق المتجر أعلى إيراد ممكن عندما يكون سعر بيع الشاشة الواحدة هو 225 ديناراً.

إيجاد أكبر زاوية

أتحقق من فهمي صفحة 129

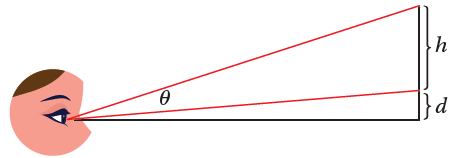نظرت سارة إلى لوحة معلقة على حائط منزلها ارتفاعها h متراً وارتفاع حافتها السفلية d متراً فوق عينها كما في الشكل المجاور. كم متراً يجب أن تبتعد سارة عن الجدار لتكون زاوية نظرها Ɵ أكبر ما يمكن؟

نسمي الأبعاد وقياسات الزوايا كما في الشكل:

$\begin{aligned} t a n θ = t a n (β - α) = \frac{t a n β - t a n α}{1 + t a n β t a n α} = \frac{\frac{h + d}{x} - \frac{d}{x}}{1 + \frac{d (h + d)}{x^{2}}}, x > 0 \\ t a n θ = \frac{x h}{x^{2} + d (h + d)} \\ s e c^{2} θ \frac{d θ}{d x} = \frac{(x^{2} + d (h + d)) (h) - x h (2 x)}{{(x^{2} + d (h + d))}^{2}} \\ \frac{d θ}{d x} = c o s^{2} x \times \frac{(- x^{2} + d (h + d)) (h)}{{(x^{2} + d (h + d))}^{2}} = 0 \end{aligned}$

بما أّن $θ < \frac{π}{2}$ ، فإن $c o s^{2} x \neq 0$ ، إذن $(- x^{2} + d (h + d)) (h) = 0$

$x^{2} = d (h + d) \to x = \sqrt{d (h + d)}$

توجد قيمة حرجة وحيدة هي: $x = \sqrt{d (h + d)}$

نستخدم اختبار المشتقة الأولى، وندرس إشارة $\frac{d θ}{d x}$

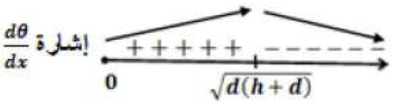
أعوض $x = \sqrt{d h}$

$\begin{aligned} \frac{d θ}{d x} & = c o s^{2} \sqrt{d h} \times \frac{(- d h + d (h + d)) (h)}{(d h + d (h + d))^{2}} \\ = c o s^{2} \sqrt{d h} \times \frac{d^{2} h}{(d h + d (h + d))^{2}} > 0 \end{aligned}$

أعوض $x = \sqrt{d (2 h + d)}$

$\begin{aligned} \frac{d θ}{d x} & = c o s^{2} \sqrt{d (2 h + d)} \times \frac{(- d (2 h + d) + d (h + d)) (h)}{(d h + d (h + d))^{2}} \\ = c o s^{2} \sqrt{d (2 h + d)} \times \frac{- d h^{2}}{(d h + d (h + d))^{2}} < 0 \end{aligned}$

إذن يجب أن تبتعد سارة عن الجدار مسافة $\sqrt{d (h + d)} m$ لتكون زاوية نظرها Ɵ أكبر ما يمكن.

تطبيقات في المستوى الإحداثي

أتحقق من فهمي صفحة 131

أجد النقطة (النقاط) الواقعة على منحنى الاقتران: $f (x) = \sqrt{8 x}$ ، التي هي أقرب ما يُمكن إلى النقطة (4, 2).

لتكن النقطة (x, y) على منحنى $f (x) = \sqrt{8 x}$ ، ولتكن المسافة بينها وبين النقطة (4, 2) هي L حيث:

$\begin{aligned} L = \sqrt{(x - 4)^{2} + (y - 2)^{2}} = \sqrt{(x - 4)^{2} + (\sqrt{8 x} - 2)^{2}} \\ \frac{d L}{d x} = \frac{x - 4 + (\sqrt{8 x} - 2) (\frac{4}{\sqrt{8 x}})}{\sqrt{(x - 4)^{2} + (\sqrt{8 x} - 2)^{2}}} = \frac{x - \frac{8}{\sqrt{8 x}}}{\sqrt{(x - 4)^{2} + (\sqrt{8 x} - 2)^{2}}} \\ \frac{d L}{d x} = 0 \to x = \frac{8}{\sqrt{8 x}} \to x \sqrt{8 x} = 8 \to 8 x^{3} = 64 \to x^{3} = 8 \to x = 2 \end{aligned}$

نستخدم اختبار المشتقة الأولى وندرس إشارة $\frac{d L}{d x}$

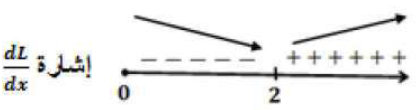
إذن أقرب نقطة من نقاط المنحنى f للنقطة (4, 2) هي: (2, 4).

إعداد : شبكة منهاجي التعليمية

25 / 11 / 2022

حمّل تطبيق منهاجي الجديد

النقاشات

حمّل تطبيق منهاجي الجديد

النقاشات

جميع الحقوق محفوظة © 2025