المحل الهندسي في المستوى المركب

أتحقق من فهمي

أتحقق من فهمي

المحل الهندسي في المستوى المركب

الدائرة

أتحقق من فهمي صفحة 169

أجد المحل الهندسي الذي تمثله المعادلة: $| z + 5 - 4 i | = 7$ ، ثم أكتب المعادلة بالصيغة الديكارتية.

$| z + 5 - 4 i | = 7 \to | z - (- 5 + 4 i) | = 7$

وهذه معادلة دائرة في المستوى المركب مركزها (-5, 4)، وطول نصف قطرها 7

$\begin{aligned} | z + 5 - 4 i | = 7 & \to | x + i y + 5 - 4 i | = 7 \\ \to | (x + 5) + (y - 4) i | = 7 \\ \to \sqrt{(x + 5)^{2} + (y - 4)^{2}} = 7 \\ \to (x + 5)^{2} + (y - 4)^{2} = 49 \end{aligned}$

وهذه معادلة دائرة مركزها (-5, 4)، وطول نصف قطرها 7

أتحقق من فهمي صفحة 171

إذا كانت: $| z + 4 - 4 \sqrt{3} i | = 4$ ، فأجيب عن السؤالين الآتيين تباعاً:

(a) أرسم المحل الهندسي الذي تمثله المعادلة في المستوى المركب.

$| z + 4 - 4 \sqrt{3} i | = 4 \to | z - (- 4 + 4 \sqrt{3} i) | = 4$

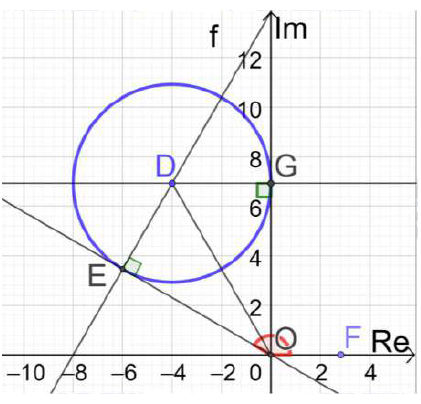
وهذه معادلة دائرة في المستوى المركب مركزها ( $\sqrt{3}$ -4, 4)، وطول نصف قطرها 4

(b) أجد القيمة العظمى لسعة الأعداد المركبة z التي تحقق المعادلة.

أكبر سعة للعدد المركب z تساوي قياس الزاوية $∠$ FOE المحصورة بين مماس الدائرة OE والمحور الحقيقي الموجب.

مماسا الدائرة OG و OE عموديان على الترتيب على نصفي القطرين DG و DE .

المثلثان OGD و OED متطابقان بثلاثة أضلاع، إذن الزاويتان $∠$ GOD و $∠$ EOD متطابقتان.

$\begin{aligned} t a n ∠ G O D = \frac{4}{4 \sqrt{3}} = \frac{1}{\sqrt{3}} \to ∠ G O D = \frac{π}{6} \\ A r g (z) = \frac{π}{2} + \frac{π}{6} + \frac{π}{6} = \frac{5 π}{6} \end{aligned}$

القيمة العظمى لسعة الأعدد المركبة z التي تحقق المعادلة المعطاة هي: $\frac{5 π}{6}$

المنصف العمودي للقطعة المستقيمة

أتحقق من فهمي صفحة 172

أجد المحل الهندسي الذي تمثله المعادلة: $| z + 1 | = | z - 5 i |$ ، ثم أكتب المعادلة بالصيغة الديكارتية.

$| z + 1 | = | z - 5 i | \to | z - (- 1) | = | z - (5 i) |$

وهذه هي معادلة المنصف العمودي للقطعة المستقيمة الواصلة بين النقطتين (0, 5)، (-1, 0).

$\begin{aligned} | z + 1 | = | z - 5 i | & \to | x + i y + 1 | = | x + i y - 5 i | \\ \to | (x + 1) + i y | = | x + i (y - 5) | \\ \to \sqrt{(x + 1)^{2} + y^{2}} = \sqrt{x^{2} + (y - 5)^{2}} \\ \to (x + 1)^{2} + y^{2} = x^{2} + (y - 5)^{2} \\ \to x^{2} + 2 x + 1 + y^{2} = x^{2} + y^{2} - 10 y + 25 \\ \to 2 x + 10 y - 24 = 0 \end{aligned}$

إذن معادلة المنصف العمودي للقطعة المستقيمة بالصيغة الديكارتية هي:

x + 5y - 12 = 0

إعداد : شبكة منهاجي التعليمية

19 / 01 / 2023

حمّل تطبيق منهاجي الجديد

النقاشات

حمّل تطبيق منهاجي الجديد

النقاشات

جميع الحقوق محفوظة © 2025