اختبار نهاية الوحدة

اختبار نهاية الوحدة

التكامل

أختار رمز الإجابة الصحيحة في كل مما يأتي:

(1) قيمة $\int \frac{x^{3} - 1}{x^{2}}$ هي:

$\frac{x^{2}}{2} - \frac{1}{x} + C$ (a

$\frac{x^{2}}{2} + \frac{1}{x} + C$ (b

$x^{2} - \frac{1}{x} + C$ (c

$x^{2} + \frac{1}{x} + C$ (d

$\begin{aligned} \int \frac{x^{3} - 1}{x^{2}} d x & = \int (\frac{x^{3}}{x^{2}} - \frac{1}{x^{2}}) d x \\ = \int (x - x^{- 2}) d x \\ = \frac{1}{2} x^{2} + x^{- 1} + C \\ = \frac{1}{2} x^{2} + \frac{1}{x} + C \dots \dots (b) \end{aligned}$

(2) إذا كان: $\int_{0}^{2} k x d x = 6$ فإن قيمة الثابت k:

1 (a

2 (b

3 (c

4 (d

$\begin{aligned} \int_{0}^{2} k x d x = 6 & {\Rightarrow \frac{k}{2} x^{2} |}_{0}^{2} = 6 \\ \Rightarrow \frac{k}{2} (2)^{2} - \frac{k}{2} (0)^{2} = 6 \\ \Rightarrow 2 k = 6 \\ \Rightarrow k = 3 \dots \dots \dots \dots \dots \dots (c) \end{aligned}$

(3) قيمة: $\int_{0}^{3} (- x^{2} + 3 x) d x$ هي:

$3 \frac{3}{4}$ (a

$21 \frac{1}{4}$ (b

$4 \frac{1}{2}$ (c

$22 \frac{1}{2}$ (d

$\begin{aligned} \int_{0}^{3} (- x^{2} + 3 x) d x & = {(- \frac{1}{3} x^{3} + \frac{3}{2} x^{2}) |}_{0}^{3} \\ = (- \frac{1}{3} (3)^{3} + \frac{3}{2} (3)^{2}) - (- \frac{1}{3} (0)^{3} + \frac{3}{2} (0)^{2}) \\ = \frac{9}{2} \dots \dots \dots \dots \dots \dots (c) \end{aligned}$

(4) قيمة: $\int_{0}^{2} e^{2 x}$ هي:

$e^{4} - 1$ (a

$e^{4} - 2$ (b

$2 e^{4} - 2$ (c

$\frac{1}{2} e^{4} - \frac{1}{2}$ (d

$\begin{aligned} \int_{0}^{2} e^{2 x} d x & = {\frac{1}{2} e^{2 x} |}_{0}^{2} \\ = \frac{1}{2} e^{2 (2)} - \frac{1}{2} e^{2 (0)} \\ = \frac{1}{2} e^{4} - \frac{1}{2} \dots \dots \dots \dots \dots \dots (d) \end{aligned}$

(5) قيمة: $\int_{1}^{4} \frac{1}{\sqrt{x}}$ هي:

2- (a

$- \frac{7}{16}$ (b

$\frac{1}{2}$ (c

2 (d

$\begin{aligned} \int_{1}^{4} \frac{1}{\sqrt{x}} d x & = \int_{1}^{4} x^{- \frac{1}{2}} d x \\ = {2 x^{\frac{1}{2}} |}_{1}^{4} \\ = {2 \sqrt{x} |}_{1}^{4} \\ = 2 \sqrt{4} - 2 \sqrt{1} \\ = 2 \dots \dots \dots \dots \dots (d) \end{aligned}$

(6) التكامل المحدود الذي يمكن عن طريقه إيجاد المساحة بين منحنى الاقتران: $f (x) = 4 x - x^{2}$ والمحور x هو:

$\int_{4}^{0} (4 x - x^{2}) d x$ (a

$\int_{0}^{4} (4 x - x^{2}) d x$ (b

$\int_{1}^{0} (4 x - x^{2}) d x$ (c

$\int_{0}^{1} (4 x - x^{2}) d x$ (d

أولاً نساوي قاعدة الاقتران بالصفر، ونحل المعادلة الآتية:

$\begin{aligned} f (x) = 0 & \Rightarrow 4 x - x^{2} = 0 \\ \Rightarrow x (4 - x) = 0 \\ \Rightarrow x = 0, x = 4 \end{aligned}$

هذه الإحداثيات تمثل حدود التكامل.

نختار عدداً ضمن الفترة [0,4]، وليكن 1 ونعوضه في قاعدة الاقتران:

$f (1) = 4 (1) - (1)^{2} = 3 > 0$

بما أن ناتج التعويض موجب، إذن منحنى الاقتران يقع فوق المحور x في الفترة [0,4]

والتكامل المحدود الذي يمكن عن طريقه إيجاد المساحة المطلوبة هو $\int_{0}^{4} (4 x - x^{2}) d x$

أجد كلاً من التكاملات الآتية:

$\int 3 x^{- 1 / 2} d x$ (7)

$\int 3 x^{- \frac{1}{2}} d x = 6 x^{\frac{1}{2}} + C$

$\int (8 x - 10 x^{2}) d x$ (8)

$\int (8 x - 10 x^{2}) d x = 4 x^{2} - \frac{10}{3} x^{3} + C$

$\int \frac{5}{x^{3}} d x$ (9)

$\begin{aligned} \int \frac{5}{x^{3}} d x & = \int 5 x^{- 3} d x \\ = - \frac{5}{2} x^{- 2} + C \\ = - \frac{5}{2 x^{2}} + C \end{aligned}$

$\int \frac{x^{2} - 1}{\sqrt[3]{x}} d x$ (10)

$\begin{aligned} \int \frac{x^{2} - 1}{\sqrt[3]{x}} d x & = \int \frac{x^{2} - 1}{x^{\frac{1}{3}}} d x \\ = \int (\frac{x^{2}}{x^{\frac{1}{3}}} - \frac{1}{x^{\frac{1}{3}}}) d x \\ = \int (x^{\frac{5}{3}} - x^{- \frac{1}{3}}) d x \\ = \frac{3}{8} x^{\frac{8}{3}} + \frac{3}{2} x^{\frac{2}{3}} + C \\ = \frac{3}{8} \sqrt[3]{x^{8}} + \frac{3}{2} \sqrt[3]{x^{2}} + C \end{aligned}$

$\int (5 x^{2} - 2 e^{7 x}) d x$ (11)

$\int (5 x^{2} - 2 e^{7 x}) d x = \frac{5}{3} x^{3} - \frac{2}{7} e^{7 x} + C$

$\int (2 x + 3 e^{4 x + 5}) d x$ (12)

$\int (2 x + 3 e^{4 x + 5}) d x = x^{2} + \frac{3}{4} e^{4 x + 5} + C$

$\int \frac{x^{2} - 6}{2 x} d x$ (13)

$\begin{aligned} \int \frac{x^{2} - 6}{2 x} d x & = \int (\frac{x^{2}}{2 x} - \frac{6}{2 x}) d x \\ = \int (\frac{1}{2} x - \frac{3}{x}) d x \\ = \frac{1}{4} x^{2} - 3 \ln | x | + C \end{aligned}$

$\int \frac{1}{(x - 1)^{3}} d x$ (14)

$\begin{aligned} \int \frac{1}{(x - 1)^{3}} d x & = \int (x - 1)^{- 3} d x \\ = - \frac{1}{2} (x - 1)^{- 2} + C \\ = - \frac{1}{2 (x - 1)^{2}} + C \end{aligned}$

$\int \frac{e^{x}}{e^{x} + 4} d x$ (15)

$\int \frac{e^{x}}{e^{x} + 4} d x = \ln | e^{x} + 4 | + C$

$\int 2 x e^{x^{2} - 1} d x$ (16)

$\begin{aligned} \int 2 x e^{x^{2} - 1} d x \\ u = x^{2} - 1 \Rightarrow \frac{d u}{d x} = 2 x \\ \Rightarrow d x = \frac{d u}{2 x} \\ \int 2 x e^{x^{2} - 1} d x = \int 2 x e^{u} \times \frac{d u}{2 x} \\ = \int e^{u} d u \\ = e^{u} + C \\ = e^{x^{2} - 1} + C \end{aligned}$

$\int 4 e^{x} (3 + e^{2 x}) d x$ (17)

$\begin{aligned} \int 4 e^{x} (3 + e^{2 x}) d x & = \int (12 e^{x} + 4 e^{3 x}) d x \\ = 12 e^{x} + \frac{4}{3} e^{3 x} + C \end{aligned}$

$\int \frac{1 + x}{{(4 + 2 x + x^{2})}^{8}} d x$ (18)

$\begin{aligned} \int \frac{1 + x}{{(4 + 2 x + x^{2})}^{8}} d x \\ u = 4 + 2 x + x^{2} \Rightarrow \frac{d u}{d x} = 2 + 2 x \\ \Rightarrow d x = \frac{d u}{2 + 2 x} \\ \int \frac{1 + x}{{(4 + 2 x + x^{2})}^{8}} d x = \int \frac{1 + x}{u^{8}} \times \frac{d u}{2 + 2 x} \\ = \int \frac{1 + x}{u^{8}} \times \frac{d u}{2 (1 + x)} \\ = \int \frac{1}{2} u^{- 8} d u \\ = - \frac{1}{14} u^{- 7} + C \\ = - \frac{1}{14} {(4 + 2 x + x^{2})}^{- 7} + C \\ = - \frac{1}{14 {(4 + 2 x + x^{2})}^{7}} + C \end{aligned}$

$\int x \sin (3 + x^{2}) d x$ (19)

$\begin{aligned} \int x \sin (3 + x^{2}) d x \\ u = 3 + x^{2} \Rightarrow \frac{d u}{d x} = 2 x \\ \Rightarrow d x = \frac{d u}{2 x} \\ \int x \sin (3 + x^{2}) d x = \int x \sin u \times \frac{d u}{2 x} \\ = \int \frac{1}{2} \sin u d u \\ = - \frac{1}{2} \cos u + C \\ = - \frac{1}{2} \cos (3 + x^{2}) + C \end{aligned}$

$\int (3 \sin 3 x - 4 \cos x) d x$ (20)

$\int (3 \sin 3 x - 4 \cos x) d x = - \cos 3 x - 4 \sin x + C$

$\int (x - \sin (7 x + 2)) d x$ (21)

$\begin{aligned} \int (x - \sin (7 x + 2)) d x & = \int_{1} x d x - \int \sin (7 x + 2) d x \\ = \frac{1}{2} x^{2} + \frac{1}{7} \cos (7 x + 2) + C \end{aligned}$

$\int (e^{3 x} - e^{- 3 x}) d x$ (22)

$\int (e^{3 x} - e^{- 3 x}) d x = \frac{1}{3} e^{3 x} + \frac{1}{3} e^{- 3 x} + C$

$\int \frac{2}{1 - 5 x} d x$ (23)

$\begin{aligned} \int \frac{2}{1 - 5 x} d x & = \int \frac{\frac{2}{- 5} (- 5)}{1 - 5 x} d x \\ = - \frac{2}{5} \int \frac{- 5}{1 - 5 x} d x \\ = - \frac{2}{5} \ln | 1 - 5 x | + C \end{aligned}$

(24) إذا كان ميل المماس لمنحنى العلاقة y هو: $\frac{d y}{d x} = 4 x - 2$ فأجد قاعدة العلاقة، علماً بان منحناها يمر بالنقطة (0,3).

$\begin{aligned} y = \int (4 x - 2) d x \\ = 2 x^{2} - 2 x + C \end{aligned}$

منحنى الاقتران يمر بالنقطة (0,3) إذن:

$\begin{aligned} 3 = 2 (0)^{2} - 2 (0) + C \\ C = 3 \\ y = 2 x^{2} - 2 x + 3 \end{aligned}$

(25) الإيراد الحدي: يمثل الاقتران: $R^{'} (x) = 4 x - 1.2 x^{2}$ الإيراد الحدي (بالدينار) لكل قطعة تباع في إحدى الشركات، حيث x عدد القطع المبيعة، و(R(x إيراد بيع x قطعة بالدينار. أجد اقتران الإيراد R(x)، علماً بأن R(20)=30000.

$\begin{aligned} R (x) & = \int (4 x - 1.2 x^{2}) d x \\ = 2 x^{2} - 0.4 x^{3} + C \end{aligned}$

بما أن R(20)=30000 إذن:

$\begin{aligned} 30000 = 2 (20)^{2} - 0.4 (20)^{3} + C \\ C = 54000 \\ R (x) = 2 x^{2} - 0.4 x^{3} + 54000 \end{aligned}$

(26) يتحرك جسيم من السكون، ويعطى تسارعه بالاقتران: $a (t) = \cos (3 t - π)$ ، حيث t الزمن بالثواني، وa تسارعه بالمتر لكل ثانية تربيع. أجد سرعة الجسيم بعد t ثانية من بدء الحركة.

$\begin{aligned} v (t) & = \int \cos (3 t - π) d x \\ = \frac{1}{3} \sin (3 t - π) + C \end{aligned}$

إذا كان $\int_{- 5}^{- 1} f (x) d x = 4, \int_{- 5}^{5} f (x) d x = 10, \int_{- 5}^{- 1} g (x) d x = 11$ ، فأجد كلاً مما يأتي:

$\int_{- 1}^{5} f (x) d x$ (27)

$\begin{aligned} \int_{- 1}^{5} f (x) d x & = \int_{- 1}^{- 5} f (x) d x + \int_{- 5}^{5} f (x) d x \\ = - 4 + 10 \\ = 6 \end{aligned}$

$\int_{- 5}^{- 1} 7 f (x) d x$ (28)

$\begin{aligned} \int_{- 5}^{- 1} 7 f (x) d x & = 7 \int_{- 5}^{- 1} f (x) d x \\ = 7 \times 4 \\ = 28 \end{aligned}$

$\int_{- 1}^{- 5} (3 f (x) - g (x)) d x$ (29)

$\begin{aligned} \int_{- 1}^{- 5} (3 f (x) - g (x)) d x & = 3 \int_{- 1}^{- 5} f (x) d x - \int_{- 1}^{- 5} g (x) d x \\ = 3 (- 4) - (- 11) \\ = - 1 \end{aligned}$

أجد قيمة كل من التكاملات الآتية:

$\int_{- 2}^{3} (3 x^{2} - 4 x + 1) d x$ (30)

$\begin{aligned} \int_{- 2}^{3} (3 x^{2} - 4 x + 1) d x & = {(x^{3} - 2 x^{2} + x) |}_{- 2}^{3} \\ = ((3)^{3} - 2 (3)^{2} + 3) - ((- 2)^{3} - 2 (- 2)^{2} - 2) \\ = 30 \end{aligned}$

$\int_{1}^{3} \frac{x^{3} + 2 x^{2}}{x} d x$ (31)

$\begin{aligned} \int_{1}^{3} \frac{x^{3} + 2 x^{2}}{x} d x & = \int_{1}^{3} (\frac{x^{3}}{x} + \frac{2 x^{2}}{x}) d x \\ = \int_{1}^{3} (x^{2} + 2 x) d x \\ = {(\frac{1}{3} x^{3} + x^{2}) |}_{1}^{3} \\ = (\frac{1}{3} (3)^{3} + (3)^{2}) - (\frac{1}{3} (1)^{3} + (1)^{2}) \\ = \frac{50}{3} \end{aligned}$

$\int_{1}^{5} | 3 - x | d x$ (32)

أعيد تعريف اقتران القيمة المطلقة:

$| 3 - x | = {\begin{cases} 3 - x, & x < 3 \\ x - 3, & x \geq 3 \end{cases}$

بما أن الاقتران تشعب عند 3، فإنني أجزئ التكامل عنده:

$\begin{aligned} \begin{aligned} \int_{1}^{5} | 3 - x | d x = \int_{1}^{3} (3 - x) d x + \int_{3}^{5} (x - 3) d x \\ = {(3 x - \frac{1}{2} x^{2}) |}_{1}^{3} + {(\frac{1}{2} x^{2} - 3 x) |}_{3}^{5} \\ = (3 (3) - \frac{1}{2} (3)^{2}) - (3 (1) - \frac{1}{2} (1)^{2}) + (\frac{1}{2} (5)^{2} - 3 (5)) - (\frac{1}{2} (3)^{2} - 3 (3)) \\ = 4 \end{aligned} \end{aligned}$

$\int_{1}^{4} \frac{20}{\sqrt{x}} d x$ (33)

$\begin{aligned} \int_{1}^{4} \frac{20}{\sqrt{x}} d x & = \int_{1}^{4} 20 x^{- \frac{1}{2}} d x \\ = {40 x^{\frac{1}{2}} |}_{1}^{4} \\ = {40 \sqrt{x} |}_{1}^{4} \\ = 40 \sqrt{4} - 40 \sqrt{1} \\ = 40 \end{aligned}$

$\int_{2}^{5} 3 x (x + 2) d x$ (34)

$\begin{aligned} \int_{2}^{5} 3 x (x + 2) d x & = \int_{2}^{5} (3 x^{2} + 6 x) d x \\ = {(x^{3} + 3 x^{2}) |}_{2}^{5} \\ = ((5)^{3} + 3 (5)^{2}) - ((2)^{3} + 3 (2)^{2}) \\ = 180 \end{aligned}$

$\int_{2}^{3} 2 x e^{- x^{2}} d x$ (35)

$\begin{aligned} \int_{2}^{3} 2 x e^{- x^{2}} d x \\ u = - x^{2} \Rightarrow & \frac{d u}{d x} = - 2 x \\ \Rightarrow d x = \frac{d u}{- 2 x} \\ x = 3 \Rightarrow u & = - 9 \\ x = 2 \Rightarrow u & = - 4 \\ \int_{2}^{3} 2 x e^{- x^{2}} d x & = \int_{- 4}^{- 9} 2 x e^{u} \times \frac{d u}{- 2 x} \\ = \int_{- 4}^{- 9} - e^{u} d u \\ = - {e^{u} |}_{- 4}^{- 9} \\ = - e^{- 9} + e^{- 4} \\ = - \frac{1}{e^{9}} + \frac{1}{e^{4}} \end{aligned}$

$\int_{0}^{2} \frac{3 x^{2}}{{(x^{3} + 1)}^{5}} d x$ (36)

$\begin{aligned} \int_{0}^{2} \frac{3 x^{2}}{{(x^{3} + 1)}^{5}} d x \\ u = x^{3} + 1 \Rightarrow \frac{d u}{d x} = 3 x^{2} \\ \Rightarrow d x = \frac{d u}{3 x^{2}} \\ x = 2 \Rightarrow u = 9 \\ x = 0 \Rightarrow u = 1 \\ \int_{0}^{2} \frac{3 x^{2}}{{(x^{3} + 1)}^{5}} d x = \int_{1}^{9} \frac{3 x^{2}}{u^{5}} \times \frac{d u}{3 x^{2}} \\ = \int_{1}^{9} u^{- 5} d u \\ = - {\frac{1}{4} u^{- 4} |}_{1}^{9} \\ = - {\frac{1}{4 u^{4}} |}_{1}^{9} \\ = - \frac{1}{4 (9)^{4}} + \frac{1}{4 (1)^{4}} \\ = \frac{1640}{6561} \end{aligned}$

$\int_{0}^{1} \frac{6 x}{x^{2} + 1} d x$ (37)

$\begin{aligned} \int_{0}^{1} \frac{6 x}{x^{2} + 1} d x & = \int_{0}^{1} \frac{3 (2 x)}{x^{2} + 1} d x \\ = 3 \int_{0}^{1} \frac{2 x}{x^{2} + 1} d x \\ = {3 \ln | x^{2} + 1 | |}_{0}^{1} \\ = 3 \ln | 2 | - 3 \ln | 1 | \\ = 3 \ln 2 \end{aligned}$

(38) إذا كان: $f (x) = {\begin{cases} x^{2} + 4 & , x < 0 \\ 4 - x & , x \geq 0 \end{cases}$ ، فأجد قيمة: $\int_{- 2}^{1} f (x) d x$ .

بما أن الاقتران تشعب عند 0، فإنني أجزئ التكامل عنده:

$\begin{aligned} \int_{- 2}^{1} f (x) d x & = \int_{- 2}^{0} (x^{2} + 4) d x + \int_{0}^{1} (4 - x) d x \\ = {(\frac{1}{3} x^{3} + 4 x) |}_{- 2}^{0} + {(4 x - \frac{1}{2} x^{2}) |}_{0}^{1} \\ = (0) - (\frac{1}{3} (- 2)^{3} + 4 (- 2)) + (4 (1) - \frac{1}{2} (1)^{2}) - (0) \\ = \frac{85}{6} \end{aligned}$

(39) يتحرك جسم في مسار مستقيم، وتعطى سرعته المتجهة بالاقتران: $v (t) = 5 + e^{t - 2}$ ، حيث t الزمن بالثواني، وv سرعته المتجهة بالمتر لكل ثانية، إذا الجسيم حركته من نقطة الأصل، فأجد موقعه بعد 3 ثوان من بدء الحركة.

$\begin{aligned} v (t) & = 5 + e^{t - 2} \\ s (t) & = \int (5 + e^{t - 2}) d t \\ = 5 t + e^{t - 2} + C \\ s (t) & = 5 t + e^{t - 2} + C \end{aligned}$

بما أن الجسيم بدأ حركته من نقطة الأصل، إذن s(0)=0:

$\begin{aligned} s (0) = 5 (0) + e^{0 - 2} + C \\ 0 = e^{- 2} + C \\ C = - e^{- 2} \\ C = - \frac{1}{e^{2}} \\ \Rightarrow s (t) = 5 t + e^{t - 2} - \frac{1}{e^{2}} \end{aligned}$

موقع الجسم بعد 3 ثوان من الحركة هو:

$\begin{aligned} s (3) & = 5 (3) + e^{3 - 2} - \frac{1}{e^{2}} \\ = 15 + e - \frac{1}{e^{2}} m \end{aligned}$

في كل مما يأتي المشتقة الأولى للاقتران (f(x، ونقطة يمر بها منحنى (y=f(x. أستعمل المعلومات المعطاة لإيجاد قاعدة الاقتران (f(x:

$f^{'} (x) = 3 x^{2} + 6 x - 2; (0, 6)$ (40)

$\begin{aligned} f (x) & = \int (3 x^{2} + 6 x - 2) d x \\ = x^{3} + 3 x^{2} - 2 x + C \end{aligned}$

بما أن منحنى الاقتران يمر بالنقطة (0,6) إذن:

$\begin{aligned} 6 = (0)^{3} + 3 (0)^{2} - 2 (0) + C \\ C = 6 \\ f (x) = x^{3} + 3 x^{2} - 2 x + 6 \end{aligned}$

$f^{'} (x) = \frac{\sqrt{20}}{x^{2}}; (1, 400)$ (41)

$\begin{aligned} f (x) & = \int \frac{\sqrt{20}}{x^{2}} d x \\ = \int \sqrt{20} x^{- 2} d x \\ = - \sqrt{20} x^{- 1} + C \\ = - \frac{\sqrt{20}}{x} + C \end{aligned}$

بما أن منحنى الاقتران يمر بالنقطة (1,400) إذن:

$\begin{aligned} 400 = - \frac{\sqrt{20}}{1} + C \\ C = 400 + \sqrt{20} \\ f (x) = - \frac{\sqrt{20}}{x} + 400 + \sqrt{20} \end{aligned}$

$f^{'} (x) = \frac{2}{x} + \frac{1}{x^{2}}; (1, 1)$ (42)

$\begin{aligned} f (x) & = \int (\frac{2}{x} + \frac{1}{x^{2}}) d x \\ = \int (\frac{2}{x} + x^{- 2}) d x \\ = 2 \ln | x | - x^{- 1} + C \\ = 2 \ln | x | - \frac{1}{x} + C \end{aligned}$

بما أن منحنى الاقتران يمر بالنقطة (1,1) إذن:

$\begin{aligned} 1 = 2 \ln | 1 | - \frac{1}{1} + C \\ C = 2 \\ f (x) = 2 \ln | x | - \frac{1}{x} + 2 \end{aligned}$

$f^{'} (x) = 5 e^{x} - 4; (0, - 1)$ (43)

$\begin{aligned} f (x) & = \int (5 e^{x} - 4) d x \\ = 5 e^{x} - 4 x + C \end{aligned}$

بما أن منحنى الاقتران يمر بالنقطة (1-,0) إذن:

$\begin{aligned} - 1 = 5 e^{0} - 4 (0) + C \\ C = - 6 \\ f (x) = 5 e^{x} - 4 x - 6 \end{aligned}$

$f^{'} (x) = x \sqrt{x^{2} + 5}; (2, 10)$ (44)

$\begin{aligned} f (x) = \int x \sqrt{x^{2} + 5} d x \\ u = x^{2} + 5 \Rightarrow \frac{d u}{d x} = 2 x \\ \Rightarrow d x = \frac{d u}{2 x} \\ \int x \sqrt{x^{2} + 5} d x = \int x u^{\frac{1}{2}} \times \frac{d u}{2 x} \\ = \int \frac{1}{2} u^{\frac{1}{2}} d u \\ = \frac{1}{3} u^{\frac{3}{2}} + C \\ = \frac{1}{3} \sqrt{{(x^{2} + 5)}^{3}} + C \end{aligned}$

بما أن منحنى الاقتران يمر بالنقطة (2,10) إذن:

$\begin{aligned} 10 = \frac{1}{3} \sqrt{{((2)^{2} + 5)}^{3}} + C \\ C = 1 \\ f (x) = \frac{1}{3} \sqrt{{(x^{2} + 5)}^{3}} + 1 \end{aligned}$

(45) أجد مساحة المنطقة المحصورة بين منحنى الاقتران: $f (x) = x^{2} - x - 2$ ، والمحور x، والمستقيمين: x=1,x=-2

أولاً نساوي قاعدة الاقتران بالصفر، ونحل المعادلة الناتجة:

$\begin{aligned} f (x) = 0 & \Rightarrow x^{2} - x - 2 = 0 \\ \Rightarrow (x + 1) (x - 2) = 0 \\ \Rightarrow x = - 1, x = 2 \end{aligned}$

نختار عدداً ضمن الفترة [2,1-]، وليكن 1.5- ونعوضه في قاعدة الاقتران:

$f (0) = (- 1.5 + 1) (- 1.5 - 2) = 1.75 > 0$

بما أن ناتج التعويض موجب، إذن منحنى الاقتران يقع فوق المحور x في الفترة [1-,2-]

نختار عددا ضمن الفترة [1,1-]، وليكن 0 ونعوضه في قاعدة الاقتران:

$f (0) = (0 + 1) (0 - 2) = - 2 < 0$

بما أن ناتج التعويض سالب، إذن منحنى الاقتران يقع تحت المحور x في الفترة [1,1-]

العدد 2 خارج الفترة المطلوبة بالسؤال، إذن نهمله

$\begin{aligned} A & = \int_{- 2}^{- 1} (x^{2} - x - 2) d x - \int_{- 1}^{1} (x^{2} - x - 2) d x \\ = \int_{- 2}^{- 1} (x^{2} - x - 2) d x + \int_{- 1}^{1} (- x^{2} + x + 2) d x \\ = {(\frac{1}{3} x^{3} - \frac{1}{2} x^{2} - 2 x) |}_{- 2}^{- 1} + {(- \frac{1}{3} x^{3} + \frac{1}{2} x^{2} + 2 x) |}_{- 1}^{1} \\ = (- \frac{1}{3} - \frac{1}{2} + 2) - (- \frac{8}{3} - 2 + 4) + (- \frac{1}{3} + \frac{1}{2} + 2) - (\frac{1}{3} + \frac{1}{2} - 2) \\ = \frac{31}{6} \end{aligned}$

إذن، المساحة هي: $\frac{31}{6}$ وحدة مربعة.

(46) طب: يمثل الاقتران (1)C تركيز دواء في الدم بعد t ساعة من حقنه في جسم مريض، حيث C مقيسة بالمليغرام لكل سنتيمتر مكعب $(mg / {cm}^{3})$ . إذا كان تركيز الدواء فـي دم المريض يتغير بمعدل $C^{'} (t) = \frac{3 t}{\sqrt{{(t^{2} + 36)}^{3}}}$ ، فأجد مقدار التغير في تركيز الدواء بالدم خلال الساعات الثماني الأولى التي تلت حقنه في جسم المريض.

أولاً نجد قاعدة الاقتران:

$\begin{aligned} C (t) = \int \frac{3 t}{\sqrt{{(t^{2} + 36)}^{3}}} d t \\ u = t^{2} + 36 \Rightarrow \frac{d u}{d t} = 2 t \\ \Rightarrow d t = \frac{d u}{2 t} \\ C (t) = \int \frac{3 t}{\sqrt{{(t^{2} + 36)}^{3}}} d t \\ = \int \frac{3 t}{\sqrt{u^{3}}} \times \frac{d u}{2 t} \\ = \int \frac{3}{2} u^{- \frac{3}{2}} d u \\ = - 3 u^{- \frac{1}{2}} + K \\ = - \frac{3}{\sqrt{u}} + K \\ = - \frac{3}{\sqrt{t^{2} + 36}} + K \end{aligned}$

بما أن مقدار تركيز الدواء في الدم في البداية هي 0 مليغرام، إذن 0=(0)C ومنه:

$\begin{aligned} C (t) = - \frac{3}{\sqrt{t^{2} + 36}} + K \\ C (0) = - \frac{3}{\sqrt{0 + 36}} + K \\ 0 = \frac{1}{2} + K \\ K = - \frac{1}{2} \\ C (t) = - \frac{3}{\sqrt{t^{2} + 36}} - \frac{1}{2} \\ C (8) = - \frac{3}{\sqrt{64 + 36}} - \frac{1}{2} = - \frac{8}{10} \end{aligned}$

مقدار التغير في تركيز الدواء في الجسم خلال الساعات الثماني الأولى من حقنه هو $- 0.8 m g / {cm}^{2}$

(47) أجد مساحة المنطقة المحصورة بين منحنى الاقتران $f (x) = 3 x^{2} - 3 x$ ، والمحور x.

أولاً نساوي قاعدة الاقتران بالصفر، ونحل المعادلة الناتجة:

$\begin{aligned} f (x) = 0 & \Rightarrow 3 x^{2} - 3 x = 0 \\ \Rightarrow 3 x (x - 1) = 0 \\ \Rightarrow x = 0, x = 1 \end{aligned}$

هذه الإحداثيات تمثل حدود التكامل.

نختار عدداً ضمن الفترة [0,1]، وليكن $\frac{1}{2}$ ونعوضه في قاعدة الاقتران:

$f (0) = 3 {(\frac{1}{2})}^{2} - 3 (\frac{1}{2}) = - \frac{3}{4} < 0$

بما أن ناتج التعويض سالب، إذن منحنى الاقتران يقع تحت المحور x في الفترة [0,1]

$\begin{aligned} A = - \int_{0}^{1} (3 x^{2} - 3 x) d x & = \int_{0}^{1} (- 3 x^{2} + 3 x) d x \\ = {(- x^{3} + \frac{3}{2} x^{2}) |}_{0}^{1} \\ = (- (1)^{3} + \frac{3}{2} (1)^{2}) - (- (0)^{3} + \frac{3}{2} (0)^{2}) \\ = \frac{1}{2} \end{aligned}$

إذن، المساحة هي: $\frac{1}{2}$ وحدة مربعة.

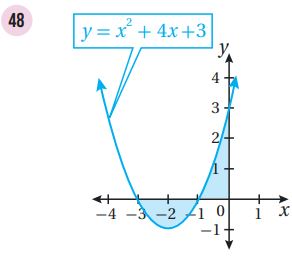
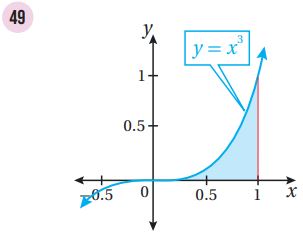
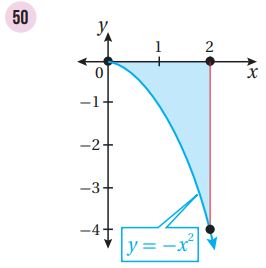
أجد مساحة المنطقة المظللة في كل من التمثيلات البيانية الآتية:

$\begin{aligned} A & = - \int_{- 3}^{- 1} (x^{2} + 4 x + 3) d x + \int_{- 1}^{0} (x^{2} + 4 x + 3) d x \\ = \int_{- 3}^{- 1} (- x^{2} - 4 x - 3) d x + \int_{- 1}^{0} (x^{2} + 4 x + 3) d x \\ = {(- \frac{1}{3} x^{3} - 2 x^{2} - 3 x) |}_{- 3}^{- 1} + {(\frac{1}{3} x^{3} + 2 x^{2} + 3 x) |}_{- 1}^{0} \\ = (\frac{1}{3} - 2 + 3) - (9 - 18 + 9) + (0) - (- \frac{1}{3} + 2 - 3) \\ = \frac{8}{3} \end{aligned}$

إذن، المساحة هي: $\frac{8}{3}$ وحدة مربعة.

$\begin{aligned} A & = \int_{0}^{1} x^{3} d x \\ = {(\frac{1}{4} x^{4}) |}_{0}^{1} \\ = (\frac{1}{4} (1)^{4}) - (\frac{1}{4} (0)^{4}) \\ = \frac{1}{4} \end{aligned}$

إذن، المساحة هي: $\frac{1}{4}$ وحدة مربعة.

$\begin{aligned} A & = - \int_{0}^{2} - x^{2} d x \\ = \int_{0}^{2} x^{2} d x \\ = {(\frac{1}{3} x^{3}) |}_{0}^{2} \\ = (\frac{1}{3} (2)^{3}) - (\frac{1}{3} (0)^{3}) \\ = \frac{8}{3} \end{aligned}$

إذن، المساحة هي: $\frac{8}{3}$ وحدة مربعة.

$\begin{aligned} A & = - \int_{- 1}^{0} x e^{x^{2}} d x + \int_{0}^{2} x e^{x^{2}} d x \\ = \int_{- 1}^{0} - x e^{x^{2}} d x + \int_{0}^{2} x e^{x^{2}} d x \\ u & = x^{2} \Rightarrow \frac{d u}{d t} = 2 x \\ \Rightarrow d t = \frac{d u}{2 x} \\ x & = 0 \Rightarrow u = 0 \\ x & = - 1 \Rightarrow u = 1 \\ x & = 2 \Rightarrow u = 4 \\ A & = \int_{1}^{0} - x e^{u} \times \frac{d u}{2 x} + \int_{0}^{4} x e^{u} \times \frac{d u}{2 x} \\ = \int_{1}^{0} - \frac{1}{2} e^{u} d u + \int_{0}^{4} \frac{1}{2} e^{u} d u \\ = {(- \frac{1}{2} e^{u}) |}_{1}^{0} + {(\frac{1}{2} e^{u}) |}_{0}^{4} \\ = (- \frac{1}{2} e^{0}) - (- \frac{1}{2} e^{1}) + (\frac{1}{2} e^{4}) - (\frac{1}{2} e^{0}) \\ = - 1 + \frac{1}{2} e + \frac{1}{2} e^{4} \end{aligned}$

إذن، المساحة هي: $- 1 + \frac{1}{2} e + \frac{1}{2} e^{4}$ وحدة مربعة.

إعداد : شبكة منهاجي التعليمية

01 / 03 / 2023

حمّل تطبيق منهاجي الجديد

النقاشات

حمّل تطبيق منهاجي الجديد

النقاشات

جميع الحقوق محفوظة © 2025