أتدرب وأحل المسائل

أتدرب وأحل المسائل

المساحات والحجوم

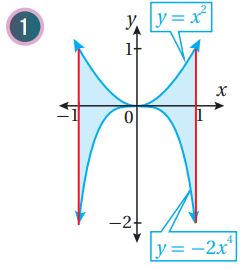
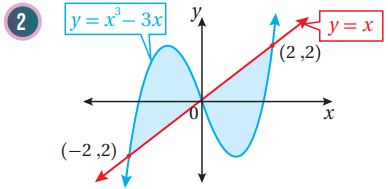
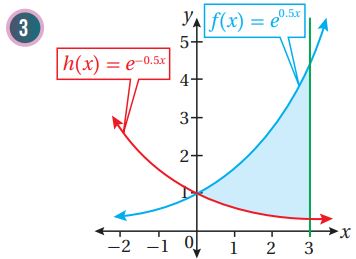
أجد مساحة المنطقة المظللة في كل من التمثيلات البيانية الآتية:

$\begin{aligned} A = \int_{- 1}^{1} (x^{2} - (- 2 x^{4})) d x & = \int_{- 1}^{1} (x^{2} + 2 x^{4}) d x \\ = {(\frac{1}{3} x^{3} + \frac{2}{5} x^{5}) |}_{- 1}^{1} \\ = (\frac{1}{3} + \frac{2}{5}) - (- \frac{1}{3} - \frac{2}{5}) \\ = \frac{22}{15} \end{aligned}$

$\begin{aligned} A & = \int_{- 2}^{0} (x^{3} - 3 x - x) d x + \int_{0}^{2} (x - (x^{3} - 3 x)) d x \\ = \int_{- 2}^{0} (x^{3} - 4 x) d x + \int_{0}^{2} (4 x - x^{3}) d x \\ = {(\frac{1}{4} x^{4} - 2 x^{2}) |}_{- 2}^{0} + {(2 x^{2} - \frac{1}{4} x^{4}) |}_{0}^{2} \\ = (0) - (4 - 8) + (8 - 4) - (0) \\ = 8 \end{aligned}$

$\begin{aligned} A = \int_{0}^{3} (e^{0.5 x} - e^{- 0.5 x}) d x & = {(2 e^{0.5 x} + 2 e^{- 0.5 x}) |}_{0}^{3} \\ = (2 e^{1.5} + 2 e^{- 1.5}) - (2 + 2) \\ = 2 e^{1.5} + 2 e^{- 1.5} - 4 \end{aligned}$

$\begin{aligned} A & = \int_{0}^{\frac{π}{4}} (\sec^{2} x - \sin x) d x \\ = {(\tan x + \cos x) |}_{0}^{\frac{π}{4}} \\ = (1 + \frac{1}{\sqrt{2}}) - (0 + 1) \\ = \frac{1}{\sqrt{2}} \end{aligned}$

(5) أجد مساحة المنطقة المحصورة بين منحنيي الاقترانين: $g (x) = 2 x^{2}, f (x) = \frac{1}{2} x^{2} + 6 :$ .

$\begin{aligned} f (x) = g (x) \Rightarrow \frac{1}{2} x^{2} + 6 = 2 x^{2} \Rightarrow \frac{3}{2} x^{2} = 6 \Rightarrow x^{2} = 4 \\ \Rightarrow x = 2, x = - 2 \\ \begin{aligned} A = \int_{- 2}^{2} (f (x) - g (x)) d x = & \int_{- 2}^{2} (\frac{1}{2} x^{2} + 6 - 2 x^{2}) d x \\ = \int_{- 2}^{2} (6 - \frac{3}{2} x^{2}) d x & = {(6 x - \frac{1}{2} x^{3}) |}_{- 2}^{2} \\ = & (12 - 4) - (- 12 + 4) \\ = & 16 \end{aligned} \end{aligned}$

(6) أجد مساحة المنطقة المحصورة بين منحنيي الاقترانين: $g (x) = 3^{x}, ، f (x) = 4^{x}$ ، والمستقيم $x = 1$ في الربع الأول.

$\begin{aligned} f (x) = g (x) ⟹ 3^{x} = 4^{x} ⟹ x = 0 \\ A = \int_{0}^{1} (f (x) - g (x)) d x = \int_{0}^{1} (4^{x} - 3^{x}) d x = {(\frac{4^{x}}{\ln 4} - \frac{3^{x}}{\ln 3}) |}_{0}^{1} \\ = (\frac{4}{\ln 4} - \frac{3}{\ln 3}) - (\frac{1}{\ln 4} - \frac{1}{\ln 3}) \\ = \frac{3}{\ln 4} - \frac{2}{\ln 3} \approx 0.344 \end{aligned}$

(7) أجد مساحة المنطقة المحصورة بين منحنيي الاقترانين: $g (x) = \cos x, f (x) = e^{x}$ ، والمستقيم $x = \frac{π}{2}$ ، في الربع الأول.

$f (x) = g (x) ⟹ e^{x} = \cos x$

نعلم من حلول هذه المعادلة الحل غير السالب: $x = 0$

في الربع الأول يكون $\cos x \leq 1$ بينما $e^{x} \geq 1$ ، إذن $e^{x} \geq \cos x$

$\begin{aligned} A = \int_{0}^{\frac{π}{2}} (e^{x} - \cos x) d x & = {(e^{x} - \sin x) |}_{0}^{\frac{π}{2}} \\ = (e^{\frac{π}{2}} - 1) - (1 - 0) \\ = e^{\frac{π}{2}} - 2 \end{aligned}$

(8) أجد المساحة المحصورة بين منحنيي الاقترانين: $g (x) = x^{4}, f (x) = | x |$ .

$\begin{aligned} g (x) = f (x) ⟹ x^{4} = | x | ⟹ x^{4} = x or x^{4} = - x \\ x^{4} = x ⟹ x^{4} - x = 0 \Rightarrow x (x^{3} - 1) = 0 ⟹ x = 0, x = 1 \\ x^{4} = - x \Rightarrow x^{4} + x = 0 \Rightarrow x (x^{3} + 1) = 0 \Rightarrow x = 0, x = - 1 \end{aligned}$

إذن، يتقاطع المنحنيان عند $x = - 1, x = 0, x = 1$ ، ويكون في الفترتين $f (x) > g (x)$

$A = \int_{- 1}^{1} (f (x) - g (x)) d x$

نجزئ هذا التكامل بسبب تغيير قاعدة $f (x)$ حول $x$ ، نحسب هذه المساحة على النحو الآتي:

$\begin{aligned} A & = \int_{- 1}^{0} (- x - x^{4}) d x + \int_{0}^{1} (x - x^{4}) d x \\ = {(- \frac{1}{2} x^{2} - \frac{1}{5} x^{5}) |}_{- 1}^{0} + {(\frac{1}{2} x^{2} - \frac{1}{5} x^{5}) |}_{0}^{1} \\ = (0) - (- \frac{1}{2} + \frac{1}{5}) + (\frac{1}{2} - \frac{1}{5}) - (0) \\ = \frac{3}{5} \end{aligned}$

(9) أجد مساحة المنطقة المحصورة بين منحنيي الاقترانين: $g (x) = - x^{2} + 2 x, f (x) = 3 x^{3} - x^{2} - 10 x$ .

$\begin{aligned} f (x) = g (x) & \Rightarrow 3 x^{3} - x^{2} - 10 x = - x^{2} + 2 x \\ \Rightarrow 3 x^{3} - 12 x = 0 \Rightarrow 3 x (x^{2} - 4) = 0 \\ \Rightarrow x = 0, x = - 2, x = 2 \end{aligned}$

بحساب قيمتي الاقترانين عند عدد بين 2- و0 مثل 1- نجد أن:

$f (- 1) = - 3 - 1 + 10 = 6, g (- 1) = - 1 - 2 = - 3$

$f (x) > g (x) \leftarrow$ في الفترة [2,0-]

بحساب قيمتي الاقترانين عند عدد بين 0 و2 مثل 1 نجد أن:

$f (1) = 3 - 1 - 10 = - 8, g (1) = - 1 + 2 = 1$

$f (x) < g (x) \leftarrow$ في الفترة [0,2]

$\begin{aligned} A & = \int_{- 2}^{0} (f (x) - g (x)) d x + \int_{0}^{2} (g (x) - f (x)) d x \\ = \int_{- 2}^{0} (3 x^{3} - x^{2} - 10 x - (- x^{2} + 2 x)) d x + \int_{0}^{2} (- x^{2} + 2 x - (3 x^{3} - x^{2} - 10 x)) d x \\ = \int_{- 2}^{0} (3 x^{3} - 12 x) d x + \int_{0}^{2} (12 x - 3 x^{3}) d x \\ = {(\frac{3}{4} x^{4} - 6 x^{2}) |}_{- 2}^{0} + {(6 x^{2} - \frac{3}{4} x^{4}) |}_{0}^{2} \\ = (0) - (12 - 24) + (24 - 12) - (0) \\ = 24 \end{aligned}$

(10) أجد مساحة المنطقة المحصورة بيـن منحنيي الاقترانين $(x) = x^{2}, f (x) = e^{x}$ ، والمستقيمين: $x = 0, x = 1$ .

$f (x) = g (x) ⟹ e^{x} = x^{2}$

يمكن استعمال الآلة الحاسبة لمعرفة أن $f (x) > g (x)$ في الفترة $[0, \infty)$

$\begin{aligned} A = \int_{0}^{1} (e^{x} - x^{2}) d x & = {(e^{x} - \frac{1}{3} x^{3}) |}_{0}^{1} \\ = (e - \frac{1}{3}) - (1 - 0) \\ = e - \frac{4}{3} \end{aligned}$

(11) أجد مساحة المنطقة المحصورة بين منحيي الاقترانين: $h (x) = 4 \sqrt{x}, f (x) = \frac{1}{2} x^{2}$ .

$\begin{aligned} f (x) = h (x) \Rightarrow \frac{1}{2} x^{2} = 4 \sqrt{x} \Rightarrow \frac{1}{4} x^{4} = 16 x \Rightarrow x^{4} - 64 x = 0 \\ \Rightarrow x (x^{3} - 64) = 0 \Rightarrow x = 0, x = 4 \end{aligned}$

$h (x) > f (x)$ في الفترة (0,4)

$\begin{aligned} A & = \int_{0}^{4} (h (x) - f (x)) d x = \int_{0}^{4} (4 \sqrt{x} - \frac{1}{2} x^{2}) d x \\ = {(\frac{8}{3} x^{\frac{3}{2}} - \frac{1}{6} x^{3}) |}_{0}^{4} = (\frac{64}{3} - \frac{32}{3}) - (0) = \frac{32}{3} \end{aligned}$

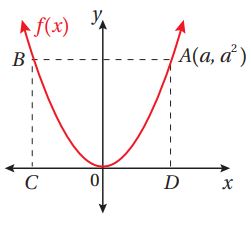
(12) يبين الشكل التالي منحنى الاقتران: $f (x) = x^{2}$ . إذا كان إحداثيا النقطة $A$ هما $A (a, a^{2})$ ، فأثبت أن مساحة المنطقة المحصورة بين منحنى الاقتران $f (x)$ والقطعة المستقيمة $\bar{A B}$ تساوي ثلثي مساحة المستطيل $A B C D$ .

من التماثل فإن $B (- a, a^{2})$

لتكن مساحة المنطقة المطلوبة:

$\begin{aligned} \int_{- a}^{a} (a^{2} - x^{2}) d x = {(a^{2} x - \frac{1}{3} x^{3}) |}_{- a}^{a} \\ = (a^{3} - \frac{1}{3} a^{3}) - (- a^{3} + \frac{1}{3} a^{3}) = 2 a^{3} - \frac{2}{3} a^{3} = \frac{4}{3} a^{3} \end{aligned}$

مساحة المستطيل ABCD هي: $2 a \times a^{2} = 2 a^{3}$

إذن، المساحة بين المنحنى والقطعة المستقيمة AB تساوي $\frac{2}{3}$ مساحة المستطيل ABCD.

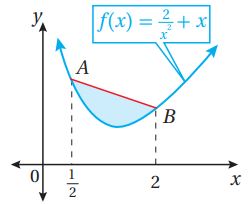
(13) يبين الشكل المجاور منحنى الاقتران: $f (x) = \frac{2}{x^{2}} + x$ . إذا كان الإحداثي $x$ لكل من النقطة $A$ والنقطة $B$ هو $\frac{1}{2}$ و $2$ على الترتيب، فأجد مساحة المنطقة المحصورة بين المستقيم $A B$ ومنحنى الاقتران $f (x)$ .

$\begin{aligned} A (\frac{1}{2}, f (\frac{1}{2})) = (\frac{1}{2}, \frac{17}{2}) \\ B (2, f (2)) = (2, \frac{5}{2}) \end{aligned}$

ميل AB:

$\frac{\frac{17}{2} - \frac{5}{2}}{\frac{1}{2} - 2} = - 4$

معادلة المستقيم AB:

$\begin{aligned} y - \frac{5}{2} = - 4 (x - 2) \\ \Rightarrow y = \frac{21}{2} - 4 x \end{aligned}$

المساحة المطلوبة هي:

$\begin{aligned} \int_{\frac{1}{2}}^{2} (\frac{21}{2} - 4 x - (2 x^{- 2} + x)) d x = \int_{\frac{1}{2}}^{2} (\frac{21}{2} - 5 x - 2 x^{- 2}) d x \\ = {(\frac{21}{2} x - \frac{5}{2} x^{2} + \frac{2}{x}) |}_{\frac{1}{2}}^{2} = 21 - 10 + 1 - (\frac{21}{4} - \frac{5}{8} + 4) = \frac{27}{8} \end{aligned}$

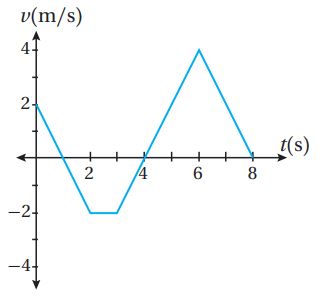
يبين الشكل المجاور منحنى السرعة المتجهة - الزمن لجسيم يتحرك على المحور $x$ في الفترة الزمنية $[0, 8]$ ، إذا بدأ الجسيم الحركة من $x = 5$ عندما $t = 0$ ، فأجد كلاً مما يأتي:

(14) إزاحة الجسيم في الفترة الزمنية المعطاة.

لتكن الإزاحة D

$D = s (8) - s (0) = \int_{0}^{8} v (t) d t = \int_{0}^{1} v (t) d t + \int_{1}^{4} v (t) d t + \int_{4}^{8} v (t) d t$

$\int_{0}^{1} v (t) d t$ يساوي مساحة المثلث الأيسر في الرسم البياني وهي:

$\frac{1}{2} (1) (2) = 1$

$\int_{1}^{4} v (t) d t$ يساوي معكوس مساحة شبه المنحرف في الرسم البياني فهو يساوي:

$- \frac{1}{2} (1 + 3) (2) = - 4$

$\int_{4}^{8} v (t) d t$ يساوي مساحة المثلث الأيمن في الرسم البياني وهي:

$\frac{1}{2} (4) (4) = 8$

إذن، إزاحة الجسيم هي: $s (8) - s (0) = 1 + (- 4) + 8 = 5 m$

(15) المسافة التي قطعها الجسيم في الفترة الزمنية المعطاة.

المسافة التي قطعها الجسيم هي: $\int_{0}^{8} | v (t) | d t$

$\begin{aligned} \int_{0}^{8} | v (t) | d t & = \int_{0}^{1} | v (t) | d t + \int_{1}^{4} | v (t) | d t + \int_{4}^{8} | v (t) | d t \\ = 1 + 4 + 8 = 13 m \end{aligned}$

(16) الموقع النهائي للجسيم.

$s (8) - s (0) = 5$

وبتعويض $s (0) = 5$ نجد أن:

$s (8) - 5 = 5 \Rightarrow s (8) = 10 m$

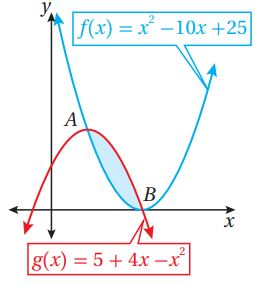
يبين الشكل المجاور منحنيي الاقترانين: $f (x) = x^{2} - 10 x + 25, g (x) = 5 + 4 x - x^{2}$ ، معتمداً هذا الشكل، أجيب عن السؤالين الآتيين تباعاً:

(17) أجد إحداثيي كل من النقطة $A$ ، والنقطة $B$ .

$\begin{aligned} f (x) = g (x) \Rightarrow x^{2} - 10 x + 25 = 5 + 4 x - x^{2} \\ \Rightarrow 2 x^{2} - 14 x + 20 = 0 \Rightarrow x^{2} - 7 x + 10 = 0 \\ \Rightarrow (x - 5) (x - 2) = 0 \Rightarrow x = 5, x = 2 \\ \Rightarrow A (2, 9), B (5, 0) \end{aligned}$

(18) أجد حجم المجسّم الناتج من دوران المنطقة المظللة حول المحور $x$ .

$\begin{aligned} V & = \int_{2}^{5} π ({(5 + 4 x - x^{2})}^{2} - {(x^{2} - 10 x + 25)}^{2}) d x \\ V & = \int_{2}^{5} π (12 x^{3} - 144 x^{2} + 540 x - 600) d x \\ = 12 π \int_{2}^{5} (x^{3} - 12 x^{2} + 45 x - 50) d x \\ = {12 π (\frac{1}{4} x^{4} - 4 x^{3} + \frac{45}{2} x^{2} - 50 x) |}_{2}^{5} \\ = 12 π (\frac{1}{4} (5)^{4} - 4 (5)^{3} + \frac{45}{2} (5)^{2} - 50 (5)) \\ - (\frac{1}{4} (2)^{4} - 4 (2)^{3} + \frac{45}{2} (2)^{2} - 50 (2)) = 81 π \end{aligned}$

(19) أجد حجم المجسّم الناتج من دوران المنطقة المحصورة بين منحنى الاقتران: $f (x) = \sqrt{\sin x}$ في الفترة $[0, π]$ ، والمحور $x$ ، حول المحور $x$ .

$\begin{aligned} V = \int_{0}^{π} π (f (x))^{2} d x = π \int_{0}^{π} \sin x d x & = - {π \cos x |}_{0}^{π} \\ = - π (\cos π - \cos 0) = 2 π \end{aligned}$

(20) أجد حجم المجسّم الناتج من دوران المنطقة المحصورة بين منحنيي الاقترانين: $g (x) = x^{3}, f (x) = \sqrt{x}$ حول المحور $x$ .

$x^{3} = \sqrt{x} \Rightarrow x^{6} = x \Rightarrow x^{6} - x = 0 \Rightarrow x (x^{5} - 1) = 0 \Rightarrow x = 0, x = 1$

لكل $x \in (0, 1)$ يكون $\sqrt{x} > x^{3}$

$\begin{aligned} V & = \int_{0}^{1} π (f^{2} (x) - g^{2} (x)) d x = π \int_{0}^{1} (x - x^{6}) d x \\ = {π (\frac{1}{2} x^{2} - \frac{1}{7} x^{7}) |}_{0}^{1} = π (\frac{1}{2} - \frac{1}{7} - 0) = \frac{5 π}{14} \end{aligned}$

(21) أجد حجم المجسم الناتج من دوران المنطقة المحصورة بين منحنى الاقتران: $f (x) = 1 + \sec x$ ، في الفترة $(- \frac{π}{2}, \frac{π}{2})$ والمستقيم $y = 3$ حول المحور $x$ .

$1 + \sec x = 3 \Rightarrow \sec x = 2 \Rightarrow \cos x = \frac{1}{2} \Rightarrow x = - \frac{π}{3}, x = \frac{π}{3}$

نلاحظ أن المنحنيين يقعان فوق المحور x وأن $f (x) = 1 + \sec x < 3$ في الفترة $(- \frac{π}{3}, \frac{π}{3})$

$\begin{aligned} \int \sec x d x = \int \sec x \times \frac{\sec x + \tan x}{\sec x + \tan x} d x \\ = \int \frac{\sec^{2} x + \sec x \tan x}{\sec x + \tan x} d x = \ln | \sec x + \tan x | + C \\ V = \int_{- \frac{π}{3}}^{\frac{π}{3}} π (9 - (1 + \sec x)^{2}) d x = π \int_{- \frac{π}{3}}^{\frac{π}{3}} (9 - (1 + 2 \sec x + \sec^{2} x)) d x \\ = π \int_{- \frac{π}{3}}^{\frac{π}{3}} (8 - 2 \sec x - \sec^{2} x) d x \\ = {π (8 x - 2 \ln | \sec x + \tan x | - \tan x) |}_{- \frac{π}{3}}^{\frac{π}{3}} \\ = π ((\frac{8 π}{3} - 2 \ln (2 + \sqrt{3}) - \sqrt{3}) - (\frac{- 8 π}{3} - 2 \ln (2 - \sqrt{3}) + \sqrt{3})) \\ = π (\frac{16 π}{3} + 2 \ln (\frac{2 - \sqrt{3}}{2 + \sqrt{3}}) - 2 \sqrt{3}) \end{aligned}$

إعداد : شبكة منهاجي التعليمية

10 / 07 / 2023

حمّل تطبيق منهاجي الجديد

النقاشات

حمّل تطبيق منهاجي الجديد

النقاشات

جميع الحقوق محفوظة © 2025