مهارات التفكير العليا

مهارات التفكير العليا

المساحات والحجوم

تبرير: أجيب عن الأسئلة الثلاثة الآتية تباعاً:

(22) أجد مساحة المنطقة المحصورة بين منحنيي الاقترانين: $y = x^{1 / 2}, \cdot y = x^{2}$ .

$\begin{aligned} x^{2} = x^{\frac{1}{2}} \Rightarrow x^{4} = x \Rightarrow x^{4} - x = 0 \Rightarrow x (x^{3} - 1) = 0 \Rightarrow x = 0, x = 1 \\ A = \int_{0}^{1} (x^{\frac{1}{2}} - x^{2}) d x = {(\frac{2}{3} x^{\frac{3}{2}} - \frac{1}{3} x^{3}) |}_{0}^{1} = \frac{2}{3} - \frac{1}{3} - 0 = \frac{1}{3} \end{aligned}$

(23) أجد المساحة المحصورة بين منحتيي الاقترانين: $y = x^{1 / 3}, \cdot y = x^{3}$ .

$\begin{aligned} x^{3} = x^{\frac{1}{3}} \Rightarrow x^{9} = x \Rightarrow x^{9} - x = 0 \Rightarrow x (x^{8} - 1) = 0 \\ \Rightarrow x (x^{4} - 1) (x^{4} + 1) = 0 \Rightarrow x (x^{2} - 1) (x^{2} + 1) (x^{4} + 1) = 0 \\ \Rightarrow x = 0, x = - 1, x = 1 \\ {(\frac{1}{8})}^{\frac{1}{3}} = \frac{1}{2}, {(\frac{1}{8})}^{3} = \frac{1}{512} \Rightarrow x^{\frac{1}{3}} > x^{3}, 0 < x < 1 \\ {(\frac{- 1}{8})}^{\frac{1}{3}} = \frac{- 1}{2}, {(\frac{- 1}{8})}^{3} = \frac{- 1}{512} \Rightarrow x^{3} > x^{\frac{1}{3}}, - 1 < x < 0 \\ A = \int_{- 1}^{0} (x^{3} - x^{\frac{1}{3}}) d x + \int_{0}^{1} (x^{\frac{1}{3}} - x^{3}) d x \\ = {(\frac{1}{4} x^{4} - \frac{3}{4} x^{\frac{4}{3}}) |}_{- 1}^{0} + {(\frac{3}{4} x^{\frac{4}{3}} - \frac{1}{4} x^{4}) |}_{0}^{1} \\ = 0 - (\frac{1}{4} - \frac{3}{4}) + \frac{3}{4} - \frac{1}{4} - 0 = 1 \end{aligned}$

(24) أجد مساحة المنطقة المحصورة بين منحنيي الاقترانين : $y = x^{1 / n}, y = x^{n}$ ، حيث $n$ عدد صحيح أكبر من أو يساوي 2، مبرراً إجابتي.

أولاً إذا كان n زوجياً

يتقاطع المنحنيان عند $x = 0, x = 1$

$\begin{matrix} A = \int_{0}^{1} (x^{\frac{1}{n}} - x^{n}) d x = {(\frac{x^{\frac{1}{n} + 1}}{\frac{1}{n} + 1} - \frac{x^{n + 1}}{n + 1}) |}_{0}^{1} = \frac{1}{\frac{1}{n} + 1} - \frac{1}{n + 1} - 0 \\ = \frac{n}{n + 1} - \frac{1}{n + 1} = \frac{n - 1}{n + 1} \end{matrix}$

ثانياً إذا كان n فردياً

يتقاطع المنحنيان عند $x = 0, x = 1, x = - 1$

$\begin{aligned} A = \int_{- 1}^{0} (x^{n} - x^{\frac{1}{n}}) d x + \int_{0}^{1} (x^{\frac{1}{n}} - x^{n}) d x \\ = {(\frac{x^{n + 1}}{n + 1} - \frac{x^{\frac{1}{n} 1}}{\frac{1}{n} + 1}) |}_{- 1}^{0} + {(\frac{x^{\frac{1}{n} + 1}}{\frac{1}{n} + 1} - \frac{x^{n + 1}}{n + 1}) |}_{0}^{1} \\ = 0 - (\frac{1}{n + 1} - \frac{1}{\frac{1}{n} + 1}) + \frac{1}{\frac{1}{n} + 1} - \frac{1}{n + 1} - 0 = \frac{- 1 + n}{n + 1} + \frac{n - 1}{n + 1} \\ = \frac{2 (n - 1)}{n + 1} \end{aligned}$

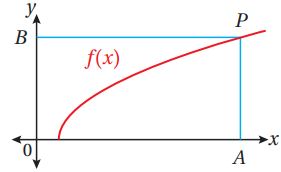تبرير: يبين الشكل المجاور منحنى الاقتران: $f (x) = \sqrt{2 x - 2}$ ، حيث: $x \geq 1$ . إذا كانت النقطة $P (9, 4)$ تقع على منحنى الاقتران $f (x)$ ، حيث $\bar{P A}$ يوازي المحور $y$ ، و $\bar{P B}$ يوازي المحور $x$ ، فأجد كلاً مما يأتي:

(25) مساحة المنطقة المحصورة بين منحنى الاقتران $f (x)$ ، والمستقيم $y = 4$ والمحورين الإحداثيين.

$\sqrt{2 x - 2} = 0 \Rightarrow x = 1$

حل 25

نقسم المنطقة المطلوب حساب مساحتها إلى قسمين برسم المستقيم x=1، ونجد المساحة كما يأتي:

$\begin{aligned} A = \int_{0}^{1} 4 d x + \int_{1}^{9} (4 - \sqrt{2 x - 2}) d x \\ = {(4 x) |}_{0}^{1} + {(4 x - \frac{1}{3} (2 x - 2)^{\frac{3}{2}}) |}_{1}^{9} \\ = 4 - 0 + 36 - \frac{1}{3} (16)^{\frac{3}{2}} - (4 - 0) = \frac{44}{3} \end{aligned}$

(26) مساحة المنطقة المحصورة بين منحنى الاقتران $f (x)$ ، والمستقيم $x = 9$ ، والمحور $x$ .

$A = \int_{1}^{9} \sqrt{2 x - 2} d x = {\frac{1}{3} (2 x - 2)^{\frac{3}{2}} |}_{1}^{9} = \frac{1}{3} ((16)^{\frac{3}{2}} - 0) = \frac{64}{3}$

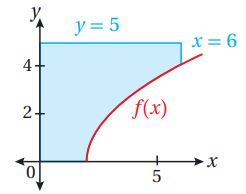(27) تبرير: بين الشكل المجاور المنطقة المحصورة بين المحورين الإحداثيين في الربع الأول، ومنحنى الاقتران: $f (x) = 2 \sqrt{x - 2}$ ، والمستقيمين: $x = 6, y = 5$ . أجد حجم المجسم الناتج من دوران المنطقة حول المحور $b b b$ ، مبرراً إجابتي.

$2 \sqrt{x - 2} = 0 \Rightarrow x = 2$

نقسم المنطقة إلى قسمين برسم المستقيم x=2، ونجد الحجم كما يأتي:

$\begin{aligned} V = π \int_{0}^{2} 5^{2} d x + π \int_{2}^{6} (5^{2} - (2 \sqrt{x - 2})^{2}) d x \\ = π \int_{0}^{2} 25 d x + π \int_{2}^{6} (25 - (4 x - 8) d x \\ = 50 π + π \int_{2}^{6} (33 - 4 x) d x = 50 π + {π (33 x - 2 x^{2}) |}_{2}^{6} \\ = 50 π + π (33 (6) - 72 - 66 + 8) ∣ \\ = 118 π \end{aligned}$

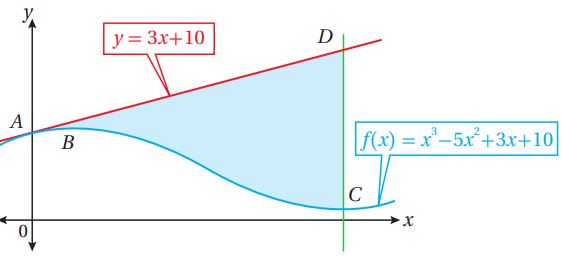تبرير: يبين الشكل المجاور منحنى كل من الاقتران: $f (x) = x^{3} - 5 x^{2} + 3 x + 10$ ، والمستقيم: $y = 3 x + 10$ . إذا مر المستقيم ومنحنى الاقتران بالنقطة $A$ الواقعة على المحور $y$ ، وكان للاقتران $f (x)$ قيمة عظمى محلية عند النقطة $B$ ، وقيمة صغرى محلية عند النقطة $C$ ، وقطع الخط الموازي للمحور $y$ والمار بالنقطة $C$ المستقيم: $y = 3 x + 10$ في النقطة $D$ ؛ فأجيب عن الأسئلة الثلاثة الآتية تباعاً:

(28) أجد إحداثيات كل من النقطة $B$ ، والنقطة $C$ .

$\begin{aligned} y = x^{3} - 5 x^{2} + 3 x + 10 \\ f^{'} (x) = 3 x^{2} - 10 x + 3 = 0 \Rightarrow (3 x - 1) (x - 3) = 0 \Rightarrow x = \frac{1}{3}, x = 3 \end{aligned}$

نقطة القيمة العظمى هي:

$B (\frac{1}{3}, f (\frac{1}{3})) = (\frac{1}{3}, \frac{283}{27})$

نقطة القيمة الصغرى هي:

$C (3, f (3)) = (3, 1)$

(29) أثبت أن $\bar{A D}$ مماس لمنحنى الاقتران $f (x)$ عند النقطة $A$ ، مبرراً إجابتي.

النقطة A تقع على محور y إذن أحداثياها هما:

$A (0, f (0)) = (0, 10)$

ميل المنحنى عند A هو:

${\frac{d y}{d x} |}_{x = 0} = 0 - 0 + 3 = 3$

معادلة مماس المنحنى $f (x)$ عند النقطة A هي (حيث $f^{'} (0) = 3$ ):

$y - 10 = 3 (x - 0) \Rightarrow y = 3 x + 10$

وهذه المعادلة هي معادلة المستقيم $\overset{\leftrightarrow}{A D}$ نفسها.

إذن، $\overset{\leftrightarrow}{A D}$ مماس لمنحنى $f (x)$ عند النقطة A

(30) أجد مساحة المنطقة المظللة، مبرراً إجابتي.

$\begin{aligned} A = & \int_{0}^{3} (3 x + 10 - (x^{3} - 5 x^{2} + 3 x + 10)) d x \\ = \int_{0}^{3} (5 x^{2} - x^{3}) d x = {(\frac{5}{3} x^{3} - \frac{1}{4} x^{4}) |}_{0}^{3} = 45 - \frac{81}{4} - 0 = \frac{99}{4} \end{aligned}$

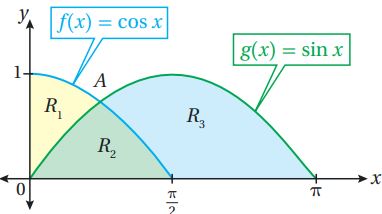تبرير: يبين الشكل المجاور منحنيي الاقترانين: $f (x) = c o s x, h (x) = s i n x$ ، معتمداً هذا الشكل، أجيب عن الأسئلة الثلاثة الآتية تباعاً:

(31) أجد إحداثيي النقطة $A$ .

$f (x) = g (x) \Rightarrow \cos x = \sin x \Rightarrow \tan x = 1 \Rightarrow x = \frac{π}{4} or x = \frac{5 π}{4}$

نلاحظ من الرسم المعطى x تقع في الفترة $(0, \frac{π}{2})$

إذن، إحداثيا النقطة A هما: $(\frac{π}{4}, f (\frac{π}{4})) = (\frac{π}{4}, \frac{1}{\sqrt{2}})$

(32) أجد مساحة كل من المناطق: $R_{1}, R_{2}, R_{3}$ .

$\begin{aligned} A (R_{1}) = \int_{0}^{\frac{π}{4}} (c o s x - s i n x) d x \\ = {(s i n x + c o s x) |}_{0}^{\frac{π}{4}} = \frac{1}{\sqrt{2}} + \frac{1}{\sqrt{2}} - (0 + 1) = \sqrt{2} - 1 \\ A (R_{2}) = \int_{0}^{\frac{π}{4}} s i n x d x + \int_{\frac{π}{4}}^{\frac{π}{2}} c o s x d x \\ = - {c o s x |}_{0}^{\frac{π}{4}} + {s i n x |}_{\frac{π}{4}}^{\frac{π}{2}} = - \frac{1}{\sqrt{2}} + 1 + 1 - \frac{1}{\sqrt{2}} = 2 - \sqrt{2} \\ A (R_{3}) = \int_{\frac{π}{4}}^{\frac{π}{2}} (s i n x - c o s x) d x + \int_{\frac{π}{2}}^{π} s i n x d x \\ = {(- c o s x - s i n x) |}_{\frac{π}{4}}^{\frac{π}{2}} + {(- c o s x) |}_{\frac{π}{2}}^{π} = \sqrt{2} \\ = - 0 - 1 - (- \frac{1}{\sqrt{2}} - \frac{1}{\sqrt{2}}) + (- (- 1) + 0) = \sqrt{2} \end{aligned}$

(33) أثبت أن مساحة المنطقة $R_{1}$ إلى مساحة المنطقة $R_{2}$ تساوي: $\sqrt{2} : 2$ .

$\frac{A (R_{1})}{A (R_{2})} = \frac{\sqrt{2} - 1}{2 - \sqrt{2}} = \frac{\sqrt{2} - 1}{\sqrt{2} (\sqrt{2} - 1)} = \frac{1}{\sqrt{2}} = \frac{\sqrt{2}}{2}$

إذن: $A (R_{1}) : A (R_{2}) = \sqrt{2} : 2$

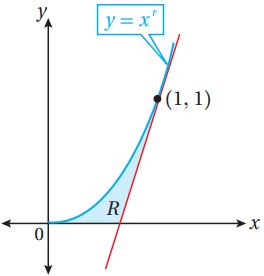تحد: يبين الشكل المجاور المنطقة $R$ المحصورة بين منحنى الاقتران: $y = x^{r}$ حيث: $r > 1$ ، والمحور $x$ ، ومماس منحنى الاقتران عند النقطة (1,1):

(34) أثبت أن مماس منحنى الاقتران يقطع المحور $x$ عند النقطة $(\frac{r - 1}{r}, 0)$ .

$y = x^{r}, y^{'} = r x^{r - 1}$

ميل المماس عند (1,1) هو:

$b b b {y^{'} |}_{x = 1} = r (1)^{r - 1} = r$

معادلة المماس هي:

$y - 1 = r (x - 1) \Rightarrow y = r x + 1 - r$

لإيجاد المقطع x لهذا المماس نضع y=0 في معادلته:

$0 = r x + 1 - r \Rightarrow x = \frac{r - 1}{r}$

إذن، يقطع هذا المماس المحور x في النقطة $(\frac{r - 1}{r}, 0)$

(35) أستعمل النتيجة من الفرع السابق لإثبات أن مساحة المنطقة $R$ هي $\frac{r - 1}{2 r (r + 1)}$ وحدة مربعة.

مساحة المنطقة R تساوي المساحة بين المنحنى والمحور x والمستقيمين x=0,x=1 مطروحاً منها مساحة المثلث الذي رؤوسه $(1, 0), (1, 1), (\frac{r - 1}{r}, 0)$ أي أن $A (R)$ هي:

$\begin{aligned} A (R) & = \int_{0}^{1} x^{r} d x - \frac{1}{2} (1 - \frac{r - 1}{r}) (1) \\ = {\frac{x^{r}}{r + 1} |}_{0}^{1} - \frac{1}{2 r} = \frac{1}{r + 1} - \frac{1}{2 r} = \frac{2 r - r - 1}{2 r (r + 1)} = \frac{r - 1}{2 r (r + 1)} \end{aligned}$

(36) أجد قيمة الثابت $r$ التي تجعل مساحة المنطقة $R$ أكبر ما يمكن.

$\begin{aligned} A (r) = \frac{r - 1}{2 r^{2} + 2 r}, r \geq 1 \\ A^{'} (r) = \frac{2 r^{2} + 2 r - (r - 1) (4 r + 2)}{{(2 r^{2} + 2 r)}^{2}} = \frac{- 2 (r^{2} - 2 r - 1)}{{(2 r^{2} + 2 r)}^{2}} = 0 \\ \Rightarrow r^{2} - 2 r - 1 = 0 \Rightarrow r = \frac{2 \pm \sqrt{8}}{2} = \frac{2 \pm 2 \sqrt{2}}{2} = 1 \pm \sqrt{2} \end{aligned}$

ولأن $r \geq 1$ تكون قيمة الحرجة $1 + \sqrt{2}$

إذن، قيمة r التي تجعل المساحة أكبر ما يمكن هي: $r = 1 + \sqrt{2}$

تحد: إذا كان العمودي على المماس لمنحنى الاقتران: $f (x) = x^{2} - 4 x + 6$ عند النقطة (1,3) يقطع منحنى الاقتران مرة أخرى عند النقطة $P$ ، فأجد كلاً مما يأتي:

(37) إحداثيات النقطة $P$ .

$f^{'} (x) = 2 x - 4$

ميل المماس عند النقطة (1,3) هو:

$f^{'} (1) = - 2$

ميل العمودي على المماس عند النقطة (1,3) هو: $\frac{1}{2}$

معادلة العمودي:

$y - 3 = \frac{1}{2} (x - 1) \Rightarrow y = \frac{1}{2} x + \frac{5}{2}$

نجد نقاط تقاطع المنحنى والعمودي على المماس:

$\begin{aligned} x^{2} - 4 x + 6 = \frac{1}{2} x + \frac{5}{2} \Rightarrow 2 x^{2} - 9 x + 7 = 0 \\ \Rightarrow (2 x - 7) (x - 1) = 0 \Rightarrow x = \frac{7}{2}, x = 1 \\ \Rightarrow P (\frac{7}{2}, f (\frac{7}{2})) = (\frac{7}{2}, \frac{17}{4}) \end{aligned}$

(38) مساحة المنطقة المحصورة بين منحنى الاقتران $f (x)$ والعمودي على المماس، مقرباً إجابتي إلى أقرب 3 منازل عشرية.

$\begin{aligned} A = \int_{1}^{\frac{7}{2}} (\frac{1}{2} x + \frac{5}{2} - (x^{2} - 4 x + 6)) d x \\ = \int_{1}^{\frac{7}{2}} (\frac{9}{2} x - \frac{7}{2} - x^{2}) d x = {(\frac{9}{4} x^{2} - \frac{7}{2} x - \frac{1}{3} x^{3}) |}_{1}^{\frac{7}{2}} \\ = (\frac{9}{4} {(\frac{7}{2})}^{2} - \frac{7}{2} (\frac{7}{2}) - \frac{1}{3} {(\frac{7}{2})}^{3}) - (\frac{9}{4} - \frac{7}{2} - \frac{1}{3}) \\ = \frac{125}{48} \approx 2.604 \end{aligned}$

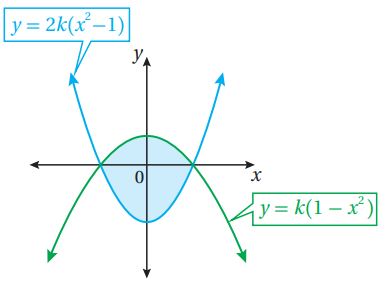(39) تبرير: المنطقة المظللة في الشكل المجاور محصورة بين قطعين مكافئين، يقطع كل منهما المحور $x$ ، عندما $x = - 1, x = 1$ . إذا كانت معادلتا القطعين هما: $y = 2 k (x^{2} - 1), y = k (1 - x^{2})$ ، وكانت مـساحة المنطقة المظللة هي 8 وحدات مربعة، فأجد قيمة الثابت $k$ .

$\begin{aligned} \int_{- 1}^{1} (k (1 - x^{2}) - 2 k (x^{2} - 1)) d x = 8 \\ \Rightarrow \int_{- 1}^{1} (k (1 - x^{2}) + 2 k (1 - x^{2})) d x = 8 \\ \Rightarrow 3 k \int_{- 1}^{1} (1 - x^{2}) d x = 8 \\ {3 k (x - \frac{1}{3} x^{3}) |}_{- 1}^{1} = 8 \\ 3 k ((1 - \frac{1}{3}) - (- 1 + \frac{1}{3})) = 8 \\ 3 k (2 - \frac{2}{3}) = 8 \\ 3 k (\frac{4}{3}) = 8 \\ \Rightarrow k = 2 \end{aligned}$

إعداد : شبكة منهاجي التعليمية

13 / 02 / 2023

حمّل تطبيق منهاجي الجديد

النقاشات

حمّل تطبيق منهاجي الجديد

النقاشات

جميع الحقوق محفوظة © 2025