مشتقتا الضرب والقسمة والمشتقات العليا

أتدرب وأحل المسائل

أتدرب وأحل المسائل

مشتقتا الضرب والقسمة والمشتقات العليا

أجد مشتقة كل اقتران ممّا يأتي:

(1) $f (x) = \frac{x^{3}}{2 x - 1}$

$\begin{array}{ll} f (x) = \frac{x^{3}}{2 x - 1} \\ f^{'} (x) = \frac{(2 x - 1) (3 x^{2}) - (x^{3}) (2)}{(2 x - 1)^{2}} = \frac{4 x^{3} - 3 x^{2}}{(2 x - 1)^{2}} \end{array}$

(2) $f (x) = x^{3} \sec x$

$\begin{aligned} f (x) & = x^{3} s e c x \\ f^{'} (x) & = (x^{3}) (s e c x t a n x) + (s e c x) (3 x^{2}) \\ = x^{3} s e c x t a n x + 3 x^{2} s e c x \end{aligned}$

(3) $f (x) = \frac{x + 1}{\cos x}$

$\begin{aligned} f (x) = \frac{x + 1}{c o s x} \\ f^{'} (x) = \frac{(c o s x) (1) - (x + 1) (- s i n x)}{(c o s x)^{2}} = \frac{c o s x + x s i n x + s i n x}{c o s^{2} x} \end{aligned}$

(4) $f (x) = e^{x} (\tan x - x)$

$\begin{aligned} f (x) & = e^{x} (t a n x - x) \\ f^{'} (x) & = (e^{x}) (s e c^{2} x - 1) + (t a n x - x) (e^{x}) \\ = e^{x} t a n^{2} x + e^{x} t a n x - x e^{x} \end{aligned}$

(5) $f (x) = \frac{\sin x + \cos x}{e^{x}}$

$\begin{array}{ll} f (x) = \frac{s i n x + c o s x}{e^{x}} \\ f^{'} (x) = \frac{(e^{x}) (c o s x - s i n x) - (s i n x + c o s x) (e^{x})}{{(e^{x})}^{2}} = \frac{- 2 s i n x}{e^{x}} \end{array}$

(6) $f (x) = x^{3} \sin x + x^{2} \cos x$

$\begin{aligned} f (x) & = x^{3} s i n x + x^{2} c o s x \\ f^{'} (x) & = (x^{3}) (c o s x) + (s i n x) (3 x^{2}) + (x^{2}) (- s i n x) + (c o s x) (2 x) \\ = x^{3} c o s x + 2 x^{2} s i n x + 2 x c o s x \end{aligned}$

(7) $f (x) = \sqrt[3]{x} (\sqrt{x} + 3)$

$\begin{array}{ll} f (x) = \sqrt[3]{x} (\sqrt{x} + 3) = x^{\frac{5}{6}} + 3 x^{\frac{1}{3}} \\ f^{'} (x) = \frac{5}{6} x^{- \frac{1}{6}} + x^{- \frac{2}{3}} = \frac{5}{6^{6}} + \frac{1}{\sqrt[3]{x^{2}}} \end{array}$

(8) $f (x) = \frac{1 + \sec x}{1 - \sec x}$

$\begin{aligned} f (x) & = \frac{1 + s e c x}{1 - s e c x} \\ f^{'} (x) & = \frac{(1 - s e c x) (s e c x t a n x) - (1 + s e c x) (- s e c x t a n x)}{(1 - s e c x)^{2}} \\ = \frac{2 s e c x t a n x}{(1 - s e c x)^{2}} \end{aligned}$

(9) $f (x) = \frac{2 - \frac{1}{x}}{x - 3}$

$\begin{aligned} f (x) = \frac{2 - \frac{1}{x}}{x - 3} = \frac{2 x - 1}{x^{2} - 3 x} \\ f^{'} (x) = \frac{(x^{2} - 3 x) (2) - (2 x - 1) (2 x - 3)}{{(x^{2} - 3 x)}^{2}} = \frac{- 2 x^{2} + 2 x - 3}{{(x^{2} - 3 x)}^{2}} \end{aligned}$

(10) $f (x) = (x^{3} - x) (x^{2} + 2) (x^{2} + x + 1)$

$\begin{aligned} f (x) = & (x^{3} - x) (x^{2} + 2) (x^{2} + x + 1) \\ f^{'} (x) = & (x^{3} - x) ((x^{2} + 2) (2 x + 1) + (x^{2} + x + 1) (2 x)) \\ + (x^{2} + 2) (x^{2} + x + 1) (3 x^{2} - 1) \\ = & (x^{3} - x) (x^{2} + 2) (2 x + 1) + (x^{3} - x) (x^{2} + x + 1) (2 x) \\ + (x^{2} + 2) (x^{2} + x + 1) (3 x^{2} - 1) \end{aligned}$

(11) $f (x) = (\csc x + \cot x)^{- 1}$

$\begin{aligned} f (x) & = (c s c x + c o t x)^{- 1} = \frac{1}{c s c x + c o t x} \\ f^{'} (x) & = \frac{- 1 (- c s c x c o t x - c s c^{2} x)}{(c s c x + c o t x)^{2}} \\ = \frac{c s c x c o t x + c s c^{2} x}{(c s c x + c o t x)^{2}} \\ = \frac{c s c x (c o t x + c s c x)}{(c s c x + c o t x)^{2}} \\ = \frac{c s c x}{c o t x + c s c x} \end{aligned}$

إذا كان f(x) و g(x) اقترانين قابلين للاشتقاق عندما x = 0 ، وكان:

$f (0) = 5, f^{'} (0) = - 3, g (0) = - 1, g^{'} (0) = 2$ فأجد كلاً ممّا يأتي:

(12) $(f g)^{'} (0)$

$\begin{aligned} (f g)^{'} (0) & = f (0) g^{'} (0) + g (0) f^{'} (0) \\ = 5 \times 2 - 1 \times - 3 = 13 \end{aligned}$

(13) ${(\frac{f}{g})}^{'} (0)$

${(\frac{f}{g})}^{'} (0) = \frac{g (0) f^{'} (0) - f (0) g^{'} (0)}{g^{2} (0)} = \frac{- 1 \times - 3 - 5 \times 2}{(- 1)^{2}} = - 7$

(14) $(7 f - 2 f g)^{'} (0)$

$(7 f - 2 f g)^{'} (0) = 7 f^{'} (0) - 2 (f g)^{'} (0) = 7 (- 3) - 2 (13) = - 47$

أجد المشتقة الثانية لكل اقتران ممّا يأتي عند قيمة x المعطاة:

(15) $f (x) = \frac{x^{2} - 4}{x^{2} + 4}, x = - 2$

$\begin{aligned} f (x) & = \frac{x^{2} - 4}{x^{2} + 4} \\ f^{'} (x) & = \frac{(x^{2} + 4) (2 x) - (x^{2} - 4) (2 x)}{{(x^{2} + 4)}^{2}} = \frac{16 x}{{(x^{2} + 4)}^{2}} \\ f^{''} (x) & = \frac{{(x^{2} + 4)}^{2} (16) - (16 x) (2) {(x^{2} + 4)}^{1} (2 x)}{{(x^{2} + 4)}^{4}} \\ = \frac{(16) (x^{2} + 4) - (16 x) (2) (2 x)}{{(x^{2} + 4)}^{3}} \\ f^{''} (- 2) & = \frac{(16) (8) - (- 32) (2) (- 4)}{(8)^{3}} = - \frac{1}{4} \end{aligned}$

(16) $f (x) = \frac{1 + x}{1 + \sqrt[3]{x}}, x = 8$

$\begin{aligned} f (x) = \frac{1 + x}{1 + \sqrt[3]{x}} = \frac{(1 + \sqrt[3]{x}) (1 - \sqrt[3]{x} + \sqrt[3]{x^{2}})}{1 + \sqrt[3]{x}} = 1 - \sqrt[3]{x} + \sqrt[3]{x^{2}} \\ f^{'} (x) = - \frac{1}{3} x^{- \frac{2}{3}} + \frac{2}{3} x^{- \frac{1}{3}} \\ f^{''} (x) = \frac{2}{9} x^{- \frac{5}{3}} - \frac{2}{9} x^{- \frac{4}{3}} = \frac{2}{9 \sqrt[3]{x^{5}}} - \frac{2}{9 \sqrt[3]{x^{4}}} \\ f^{''} (8) = \frac{2}{9 \sqrt[3]{8^{5}}} - \frac{2}{9 \sqrt[3]{8^{4}}} = \frac{2}{9} (\frac{1}{32} - \frac{1}{16}) = - \frac{1}{144} \end{aligned}$

(17) $f (x) = \frac{1}{1 + \sqrt{x}}, x = 4$

$\begin{aligned} f (x) & = \frac{1}{1 + \sqrt{x}} \\ f^{'} (x) & = \frac{- (\frac{1}{2 \sqrt{x}})}{(1 + \sqrt{x})^{2}} = \frac{- 1}{2 \sqrt{x} (1 + \sqrt{x})^{2}} \\ f^{''} (x) & = \frac{2 \sqrt{x} (2) (1 + \sqrt{x})^{1} (\frac{1}{2 \sqrt{x}}) + (1 + \sqrt{x})^{2} (\frac{1}{\sqrt{x}})}{4 x (1 + \sqrt{x})^{4}} \\ = \frac{2 + \frac{1 + \sqrt{x}}{\sqrt{x}}}{4 x (1 + \sqrt{x})^{3}} \\ f^{''} (4) & = \frac{2 + \frac{1 + 2}{2}}{16 (1 + 2)^{3}} = \frac{7}{864} \end{aligned}$

أجد معادلة المماس لكل اقتران ممّا يأتي عند النقطة المعطاة:

(18) $f (x) = \frac{1 + x}{1 + e^{x}}, (0, \frac{1}{2})$

$\begin{aligned} f (x) = \frac{1 + x}{1 + e^{x}} \\ f^{'} (x) = \frac{(1 + e^{x}) (1) - (1 + x) (e^{x})}{{(1 + e^{x})}^{2}} = \frac{1 - x e^{x}}{{(1 + e^{x})}^{2}} \end{aligned}$

ميل المماس عند النقطة (0, $\frac{1}{2}$ ) هو: $\frac{1}{4}$ f ’(0) =

معادلة المماس هي:

$y - \frac{1}{2} = \frac{1}{4} (x - 0) \to y = \frac{1}{4} x + \frac{1}{2}$

(19) $f (x) = e^{x} \cos x + \sin x, (0, 1)$

$\begin{aligned} f (x) = e^{x} c o s x + s i n x \\ f^{'} (x) = (e^{x}) (- s i n x) + (c o s x) (e^{x}) + c o s x \end{aligned}$

ميل المماس عند النقطة (0, 1) هو:

$f^{'} (0) = (1) (0) + (1) (1) + 1 = 2$

معادلة المماس هي:

$y - 1 = 2 (x - 0) \to y = 2 x + 1$

أثبت صحّة كلّ ممّا يأتي معتمداً أنّ $\frac{d}{d x} (\cos x) = - \sin x \cdot \frac{d}{d x} (\sin x) = \cos x$ :

(20) $\frac{d}{d x} (\cot x) = - \csc^{2} x$

$\begin{aligned} \frac{d}{d x} (c o t x) & = \frac{d}{d x} (\frac{c o s x}{s i n x}) \\ = \frac{(s i n x) (- s i n x) - (c o s x) (c o s x)}{s i n^{2} x} \\ = \frac{- s i n^{2} x - c o s^{2} x}{s i n^{2} x} \\ = - \frac{1}{s i n^{2} x} \\ = - c s c^{2} x \end{aligned}$

(21) $\frac{d}{d x} (\sec x) = \sec x \tan x$

$\begin{aligned} \frac{d}{d x} (s e c x) & = \frac{d}{d x} (\frac{1}{c o s x}) \\ = \frac{- (- s i n x)}{c o s^{2} x} \\ = \frac{1}{c o s x} \times \frac{s i n x}{c o s x} \\ = s e c x t a n x \end{aligned}$

(22) $\frac{d}{d x} (\csc x) = - \csc x \cot x$

$\begin{aligned} \frac{d}{d x} (c s c x) & = \frac{d}{d x} (\frac{1}{s i n x}) \\ = \frac{- (c o s x)}{s i n^{2} x} \\ = - \frac{1}{s i n x} \times \frac{c o s x}{s i n x} \\ = - c s c x c o t x \end{aligned}$

ألاحظ المشتقة المعطاة في كلّ ممّا يأتي، ثم أجد المشتقة العليا المطلوبة:

(23) $f^{''} (x) = 2 - \frac{2}{x}, f^{'''} (x)$

$\begin{aligned} f^{''} (x) = 2 - \frac{2}{x} \\ f^{'''} (x) = \frac{2}{x^{2}} \end{aligned}$

(24) $f^{'''} (x) = 2 \sqrt{x}, f^{(4)} (x)$

$\begin{aligned} f^{'''} (x) = 2 \sqrt{x} \\ f^{(4)} (x) = \frac{1}{\sqrt{x}} \end{aligned}$

(25) $f^{(4)} (x) = 2 x + 1, f^{(6)} (x)$

$\begin{aligned} f^{(4)} (x) = 2 x + 1 \\ f^{(5)} (x) = 2 \\ f^{(6)} (x) = 0 \end{aligned}$

$نباتات هجينة$ (26) نباتات هجينة: وجد فريق بحث زراعي أنّه يمكن التعبير عن ارتفاع نبتة هجينة من نبات تبّاع الشمس h بالأمتار، باستعمال الاقتران: h(t) = $\frac{3 t^{2}}{4 + t^{2}}$ ، حيث t الزمن بالأشهر بعد زراعة البذور. أجد معدّل تغير ارتفاع النبتة بالنسبة إلى الزمن.

$\begin{aligned} h (t) = \frac{3 t^{2}}{4 + t^{2}} \\ h^{'} (t) = \frac{(4 + t^{2}) (6 t) - (3 t^{2}) (2 t)}{{(4 + t^{2})}^{2}} = \frac{24 t}{{(4 + t^{2})}^{2}} \end{aligned}$

إذا كان الاقتران: y = e^x sin x ، فأجيب عن السؤالين الآتيين تباعاً:

(27) أجد $\frac{d y}{d x}$ ، و $\frac{d^{2} y}{d x^{2}}$ .

$\begin{aligned} \frac{d y}{d x} = (e^{x}) (c o s x) + (s i n x) (e^{x}) = e^{x} (c o s x + s i n x) \\ \frac{d^{2} y}{d x^{2}} = e^{x} (- s i n x + c o s x) + e^{x} (c o s x + s i n x) = 2 e^{x} c o s x \end{aligned}$

(28) أثبت أنّ $\frac{d^{2} y}{d x^{2}} = 2 \frac{d y}{d x} - 2 y$

$\begin{aligned} 2 \frac{d y}{d x} - 2 y & = 2 e^{x} (c o s x + s i n x) - 2 e^{x} s i n x \\ = 2 e^{x} c o s x \\ = \frac{d^{2} y}{d x^{2}} \end{aligned}$

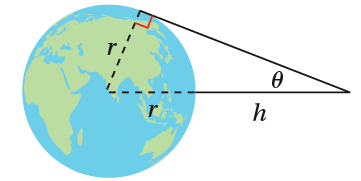أقمار صناعية: عندما ترصد الأقمار الصناعية الأرض، فإنه يُمكنها مسح جزء فقط من سطح الأرض. وبعض الأقمار الصناعية تحوي مُستشعرات لقياس الزاوية Ɵ (بالراديان) المبينة في الشكل المجاور. إذا كان h يمثل المسافة بين القمر الصناعي وسطح الأرض بالكيلومترات، و r يُمثل نصف قطر الأرض بالكيلومترات، فأجيب عن السؤالين الآتيين تباعاً:

(29) أثبت أنّ h = r(csc Ɵ - 1) .

$\begin{aligned} c s c θ = \frac{r + h}{r} & \to r + h = r c s c θ \\ \to h = r (c s c θ - 1) \end{aligned}$

(30) أجد معدل تغير h بالنسبة إلى Ɵ عندما $θ = \frac{π}{6} rad$ (أفترض أن r = 6371 km).

$\begin{aligned} \frac{d h}{d θ} & = r (- c s c θ c o t θ) \\ {\frac{d h}{d θ} |}_{θ = \frac{π}{6}} & = 6371 (- c s c \frac{π}{6} c o t \frac{π}{6}) \\ = 6371 (- 2 \times \sqrt{3}) \approx - 22070 km / rad \end{aligned}$

(31) إذا كان: $f (x) = 9 \ln x + \frac{1}{2 x^{2}}$ ، فأثبت أنّ $f^{'} (x) = \frac{(3 x - 1) (3 x + 1)}{x^{3}}$ .

$\begin{aligned} f (x) & = 9 l n x + \frac{1}{2 x^{2}} \\ f^{'} (x) & = 9 (\frac{1}{x}) + \frac{- 1 (4 x)}{4 x^{4}} \\ = \frac{9}{x} - \frac{1}{x^{3}} \\ = \frac{9 x^{2} - 1}{x^{3}} \\ = \frac{(3 x - 1) (3 x + 1)}{x^{3}} \end{aligned}$

يبين الشكل المجاور منحنيي الاقتراني: F(x) ، و G(x) .

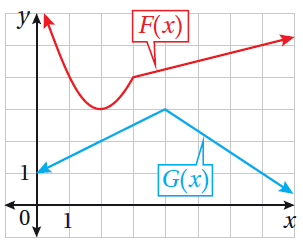
إذا كان: P(x) = F(x)G(x) ، وكان: $\frac{F (x)}{G (x)}$ Q(x) = ، فأجد كلاً ممّا يأتي:

(32) P’(2)

$P^{'} (2) = F (2) G^{'} (2) + G (2) F^{'} (2)$

(2) G’ ميل المستقيم الذي يمر بالنقطتين (2, 2) و (4, 3) ويساوي

(2) F’ ميل المماس الأفقي، ويساوي صفراً.

$P^{'} (2) = 3 \times \frac{1}{2} + 2 \times 0 = \frac{3}{2}$

(33) Q’(7)

$Q^{'} (7) = \frac{G (7) F^{'} (7) - F (7) G^{'} (7)}{G^{2} (7)} = \frac{1 \times \frac{1}{4} - 5 \times - \frac{2}{3}}{1} = \frac{43}{12}$

إعداد : شبكة منهاجي التعليمية

21 / 10 / 2022

النقاشات

جميع الحقوق محفوظة © 2024