أستعد لدراسة الوحدة
المعدلات المرتبطة
حل المثلث باستعمال قانون جيوب التمام
أجد قيمة x في كلّ من المثلثات الآتية:
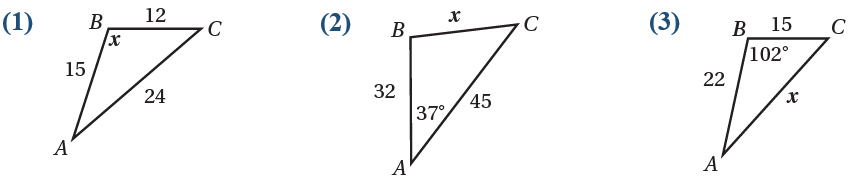
(1)
(2)
(3)
حل المعادلات المثلثية
أحل كل معادلة ممّا يأتي في الفترة [0, 2π) :
(4) tan 2x + 1 = 0
(5) 2sin2 x + sin x = 0
(6) 1 – cos x =
تحديد فترات التزايد وفترات التناقص
أحدد فترات التزايد وفترات التناقص لكل اقتران ممّا يأتي:
(7) f(x) = 6x2 – 6x + 12
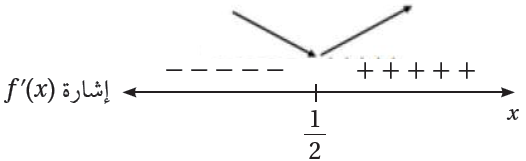
الاقتران f متناقص في (-∞, ) ومتزايد في (
, ∞).
(8) f(x) = x3 – 3x2 + 4x + 3
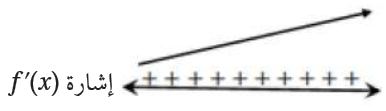
ليس للمشتقة أصفار وإشارتها مماثلة لإشارة معامل x2 لجميع الأعداد الحقيقية؛ أي أنّ:
f ’ (x) > 0 ؛ فالاقتران متزايد على R
(9) f(x) = x2 – 8x2

الاقتران f متزايد على (-∞ , ) و (0
, ).
الاقتران f متناقص على (, 0) و (
, ∞).
إعداد : شبكة منهاجي التعليمية
26 / 12 / 2022
النقاشات