أتدرب وأحل المسائل

أتدرب وأحل المسائل

المساحة

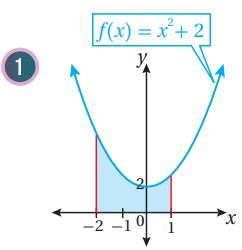
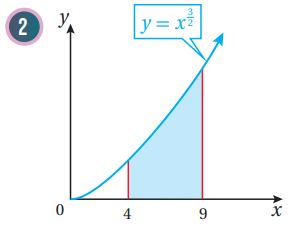
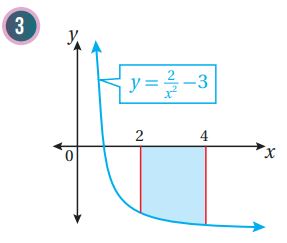
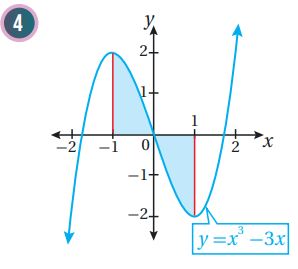
أجد مساحة المنطقة المظللة في كل من التمثيلات البيانية الآتية:

$\begin{aligned} A = \int_{- 2}^{1} (x^{2} + 2) d x & = {(\frac{1}{3} x^{3} + 2 x) |}_{- 2}^{1} \\ = (\frac{1}{3} (1)^{3} + 2 (1)) - (\frac{1}{3} (- 2)^{3} + 2 (- 2)) \\ = 9 \end{aligned}$

$\begin{aligned} A = \int_{4}^{9} x^{\frac{3}{2}} d x & = {(\frac{2}{5} x^{\frac{5}{2}}) |}_{4}^{9} \\ = {(\frac{2}{5} \sqrt[2]{x^{5}}) |}_{4}^{9} \\ = (\frac{2}{5} \sqrt[2]{9^{5}}) - (\frac{2}{5} \sqrt[2]{4^{5}}) \\ = \frac{422}{5} \end{aligned}$

$\begin{aligned} A = - \int_{2}^{4} (\frac{2}{x^{2}} - 3) d x & = - \int_{2}^{4} (2 x^{- 2} - 3) d x \\ = \int_{2}^{4} (- 2 x^{- 2} + 3) d x \\ = {(2 x^{- 1} + 3 x) |}_{2}^{4} \\ = {(\frac{2}{x} + 3 x) |}_{2}^{4} \\ = (\frac{2}{4} + 3 (4)) - (\frac{2}{2} + 3 (2)) \\ = \frac{11}{2} \end{aligned}$

$\begin{aligned} A & = \int_{- 1}^{0} (x^{3} - 3 x) d x - \int_{0}^{1} (x^{3} - 3 x) d x \\ = \int_{- 1}^{0} (x^{3} - 3 x) d x + \int_{0}^{1} (- x^{3} + 3 x) d x \\ = {(\frac{1}{4} x^{4} - \frac{3}{2} x^{2}) |}_{- 1}^{0} + {(- \frac{1}{4} x^{4} + \frac{3}{2} x^{2}) |}_{0}^{1} \\ = (0) - (\frac{1}{4} (- 1)^{4} - \frac{3}{2} (- 1)^{2}) + (- \frac{1}{4} (1)^{4} + \frac{3}{2} (1)^{2}) - (0) \\ = \frac{5}{2} \end{aligned}$

$\begin{aligned} A = \int_{0}^{3} (x + 1) d x & = {(\frac{1}{2} x^{2} + x) |}_{0}^{3} \\ = (\frac{1}{2} (3)^{2} + 3) - (\frac{1}{2} (0)^{2} + 0) \\ = \frac{15}{2} \end{aligned}$

$\begin{aligned} A = \int_{0}^{2} 3 x^{2} d x & = {x^{3} |}_{0}^{3} \\ = (3^{3}) - (0^{3}) \\ = 27 \end{aligned}$

(7) أجد مساحة المنطقة المحصورة بين منحنى الاقتران: $f (x) = 3 x^{2} - 2 x + 2$ ، والمحور $x$ ، والمستقيمين: $x = 0, x = 2$

$f (x) = 3 x^{2} - 2 x + 2$

أولاً نساوي قاعدة الاقتران بالصفر، ونحل المعادلة الناتجة:

$f (x) = 0 \Rightarrow 3 x^{2} - 2 x + 2 = 0$

نحسب المميز:

$Δ = b^{2} - 4 a c = (- 2)^{2} - 4 (3) (2) = - 20$

بما أن المميز سالب، إذن لا يوجد حلول لهذه المعادلة، وتكون حدود التكامل هي 0 و 2 نختار عدداً ضمن الفترة [0,2]، وليكن 1 ونعوضه في قاعدة الاقتران:

$f (1) = 3 (1)^{2} - 2 (1) = 1 > 0$

بما أن ناتج التعويض موجب، إذن منحنى الاقتران يقع فوق المحور x في الفترة [2,0]

$\begin{aligned} A = \int_{0}^{2} (3 x^{2} - & 2 x + 2) d x = {(\frac{1}{3} x^{3} - x^{2} + 2 x) |}_{0}^{2} \\ = (\frac{1}{3} (2)^{3} - (2)^{2} + 2 (2)) - (\frac{1}{3} (0)^{3} - (0)^{2} + 2 (0)) \\ = \frac{8}{3} \end{aligned}$

إذن، المساحة هي: $\frac{8}{3}$ وحدة مربعة.

(8) جد مساحة المنطقة المحصورة بين منحنى الاقتران: $f (x) = 9 - x^{2}$ ، والمحور $x$

$f (x) = 9 - x^{2}$

أولاً نساوي قاعدة الاقتران بالصفر، ونحل المعادلة الناتجة:

$\begin{aligned} f (x) = 0 & \Rightarrow 9 - x^{2} = 0 \\ \Rightarrow (3 + x) (3 - x) = 0 \\ \Rightarrow x = - 3, x = 3 \end{aligned}$

هذه الإحداثيات تمثل حدود التكامل.

نختار عدداً ضمن الفترة [3,3-]، وليكن 0 ونعوضه في قاعدة الاقتران:

$f (0) = 9 - (0)^{2} = 9 > 0$

بما أن ناتج التعويض موجب، إذن منحنى الاقتران يقع فوق المحور x في الفترة [3,3-]

$\begin{aligned} A = \int_{- 3}^{3} (9 - x^{2}) d x & = {(9 x - \frac{1}{3} x^{3}) |}_{- 3}^{3} \\ = (9 (3) - \frac{1}{3} (3)^{3}) - (9 (- 3) - \frac{1}{3} (- 3)^{3}) \\ = 36 \end{aligned}$

إذن، المساحة هي: 36 وحدة مربعة.

(9) أجد مساحة المنطقة المحصورة بين منحنى الاقتران: $f (x) = x^{3} + 4 x$ ، والمحور $x$ ، والمستقيمين: $x = - 1, x = 2$

$f (x) = x^{3} + 4 x$

أولاً نساوي قاعدة الاقتران بالصفر، ونحل المعادلة الناتجة:

$\begin{aligned} f (x) = 0 & \Rightarrow x^{3} + 4 x = 0 \\ \Rightarrow x (x^{2} + 4) = 0 \\ \Rightarrow x = 0 \end{aligned}$

مميز العبارة التربيعية $(x^{2} + 4)$ سالب، لذا لا أصفار لها.

نختار عدداً ضمن الفترة [1,0-]، وليكن $- \frac{1}{2}$ ونعوضه في قاعدة الاقتران:

$f (- \frac{1}{2}) = {(- \frac{1}{2})}^{3} + 4 (- \frac{1}{2}) = - \frac{17}{2} < 0$

بما أن ناتج التعويض سالب، إذن منحنى الاقتران يقع تحت المحور x في الفترة [1,0-]

نختار عدداً ضمن الفترة [0,2]، وليكن 1 ونعوضه في قاعدة الاقتران:

$f (1) = (1)^{3} + 4 (1) = 5 > 0$

بما أن ناتج التعويض موجب، إذن منحنى الاقتران يقع فوق المحور x في الفترة [0,2]

$\begin{aligned} A & = - \int_{- 1}^{0} (x^{3} + 4 x) d x + \int_{0}^{2} (x^{3} + 4 x) d x \\ = \int_{- 1}^{0} (- x^{3} - 4 x) d x + \int_{0}^{2} (x^{3} + 4 x) d x \\ = {(- \frac{1}{4} x^{4} - 2 x^{2}) |}_{- 1}^{0} + {(\frac{1}{4} x^{4} + 2 x^{2}) |}_{0}^{2} \\ = ((0) - (- \frac{1}{4} (- 1)^{4} - 2 (- 1)^{2})) + ((- \frac{1}{4} (2)^{4} + 2 (2)^{2}) - (0)) \\ = \frac{25}{4} \end{aligned}$

إذن، المساحة هي: $\frac{25}{4}$ وحدة مربعة.

(10) أجد مساحة المنطقة المحصورة بين منحنى الاقتران: $f (x) = - 7 + 2 x - x^{2}$ ، والمحور $x$ ، والمستقيمين: $x = 1, x = 4$

$f (x) = - 7 + 2 x - x^{2}$

أولاً نساوي قاعدة الاقتران بالصفر، ونحل المعادلة الناتجة:

$f (x) = 0 \Rightarrow - 7 + 2 x - x^{2} = 0$

نحسب المميز:

$Δ = b^{2} - 4 a c = (2)^{2} - 4 (- 1) (- 7) = - 24$

بما أن المميز سالب، إذن لا يوجد حلول لهذه المعادلة، وتكون حدود التكامل هي 1 و 4 نختار عدداً ضمن الفترة [1,4]، وليكن 2 ونعوضه في قاعدة الاقتران:

$f (1) = - 7 + 2 (1) - (1)^{2} = - 6 < 0$

بما أن ناتج التعويض سالب، إذن منحنى الاقتران يقع تحت المحور x في الفترة [1,4]

$\begin{aligned} A & = - \int_{1}^{4} (- 7 + 2 x - x^{2}) d x \\ = \int_{1}^{4} (7 - 2 x + x^{2}) d x \\ = {(7 x - x^{2} + \frac{1}{3} x^{3}) |}_{1}^{4} \\ = (7 (4) - (4)^{2} + \frac{1}{3} (4)^{3}) - (7 (1) - (1)^{2} + \frac{1}{3} (1)^{3}) \\ = 27 \end{aligned}$

إذن، المساحة هي: 27 وحدة مربعة.

(11) أجد مساحة المنطقة المحصورة بين منحنى الاقتران: $f (x) = 5 - x$ ، والمحور $x$ ، والمستقيمين: $x = 3, x = 5$

$f (x) = 5 - x$

أولاً نساوي قاعدة الاقتران بالصفر، ونحل المعادلة الناتجة:

$\begin{aligned} f (x) = 0 & \Rightarrow 5 - x = 0 \\ \Rightarrow x = 5 \end{aligned}$

نختار عدداً ضمن الفترة [3,5]، وليكن 4 ونعوضه في قاعدة الاقتران:

$f (4) = 5 - (4) = 1 > 0$

بما أن ناتج التعويض موجب، إذن منحنى الاقتران يقع فوق المحور x في الفترة [3,5]

$\begin{aligned} A = \int_{3}^{5} (5 - x) d x & = {(5 x - \frac{1}{2} x^{2}) |}_{3}^{5} \\ = ((5 (5) - \frac{1}{2} (5)^{2}) - (5 (3) - \frac{1}{2} (3)^{2})) \\ = 2 \end{aligned}$

إذن، المساحة هي: 2 وحدة مربعة.

(12) أجد مساحة المنطقة المحصورة بين منحنى الاقتران: $f (x) = (x + 1) (x - 4)$ ، والمحور $x$

$f (x) = (x + 1) (x - 4)$

أولاً نساوي قاعدة الاقتران بالصفر، ونحل المعادلة الناتجة:

$\begin{aligned} f (x) = 0 & \Rightarrow (x + 1) (x - 4) = 0 \\ \Rightarrow x = - 1, x = 4 \end{aligned}$

هذه الإحداثيات تمثل حدود التكامل.

نختار عدداً ضمن الفترة [1,4-]، وليكن 0 ونعوضه في قاعدة الاقتران:

$f (0) = (0 + 1) (0 - 4) = - 4 < 0$

بما أن ناتج التعويض سالب، إذن منحنى الاقتران يقع تحت المحور x في الفترة [1,4-]

$\begin{aligned} A = - \int_{- 1}^{4} (x + 1) (x - 4) d x = - \int_{- 1}^{4} (x^{2} + x - 4 x - 4) d x \\ = - \int_{- 1}^{4} (x^{2} - 3 x - 4) d x \\ = \int_{- 1}^{4} (- x^{2} + 3 x + 4) d x \\ = {(- \frac{1}{3} x^{3} + \frac{3}{2} x^{2} + 4 x) |}_{- 1}^{4} \\ = (- \frac{1}{3} (4)^{3} + \frac{3}{2} (4)^{2} + 4 (4)) - (- \frac{1}{3} (- 1)^{3} + \frac{3}{2} (- 1)^{2} + 4 (- 1)) \\ = \frac{125}{6} \end{aligned}$

إذن، المساحة هي: $\frac{25}{6}$ وحدة مربعة.

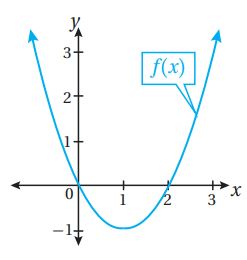
يبين الشكل المجاور منحنى الاقتران $f (x) = x^{2} - 2 x$ :

(13) أجد مساحة المنطقة المحصورة بين منحنى الاقتران، والمحور $x$

$f (x) = x^{2} - 2 x$

حسب الشكل، فإن منحنى الاقتران يقع تحت المحور x في الفترة [0,2]

$\begin{aligned} A = - \int_{0}^{2} (x^{2} - 2 x) d x = \int_{0}^{2} (- x^{2} + 2 x) d x \\ = {(- \frac{1}{3} x^{3} + x^{2}) |}_{0}^{2} \\ = (- \frac{1}{3} (2)^{3} + (2)^{2}) - (- \frac{1}{3} (0)^{3} + (0)^{2}) \\ = \frac{4}{3} \end{aligned}$

إذن، المساحة هي: $\frac{4}{3}$ وحدة مربعة.

(14) أجد مساحة المنطقة المحصورة بين منحنى الاقتران، والمحور $x$ ، والمستقيم $x = 3$

$\begin{aligned} A & = \int_{2}^{3} (x^{2} - 2 x) d x \\ = {(\frac{1}{3} x^{3} - x^{2}) |}_{2}^{3} \\ = ((9 - 9) - (\frac{8}{3} - 4)) \\ = \frac{4}{3} \end{aligned}$

إذن، المساحة هي: $\frac{4}{3}$ وحدة مربعة.

(15) أجد مساحة المنطقة المحصورة بين منحنى الاقتران، والمحور $x$ ، والمستقيم $x = - 1$

$\begin{aligned} A & = \int_{- 1}^{0} (x^{2} - 2 x) d x \\ = {(\frac{1}{3} x^{3} - x^{2}) |}_{- 1}^{0} \\ = (0) - (- \frac{1}{3} - 1) \\ = \frac{4}{3} \end{aligned}$

إذن، المساحة هي: $\frac{4}{3}$ وحدة مربعة.

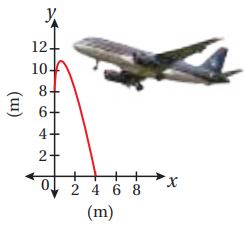
(16) يبين التمثيل البياني المجاور شكل السطح العلوي لجناح طائرة، ممثلاً بالمعادلة: $y = 8 + 8 \sqrt{x} - 6 x$ ، حيث: $0 \leq x \leq 4$ . أجد مساحة السطح العلوي لجناح الطائرة.

$\begin{aligned} A = \int_{0}^{4} (8 + 8 \sqrt{x} - 6 x) d x & = \int_{0}^{4} (8 + 8 x^{\frac{1}{2}} - 6 x) d x \\ = {(8 x + \frac{16}{3} x^{\frac{3}{2}} - 3 x^{2}) |}_{0}^{4} \\ = {(8 x + \frac{16}{3} \sqrt{x^{3}} - 3 x^{2}) |}_{0}^{4} \\ = (8 (4) + \frac{16}{3} \sqrt{4^{3}} - 3 (4)^{2}) - (0) \\ = \frac{80}{3} \end{aligned}$

إذن، المساحة هي: $\frac{80}{3}$ وحدة مربعة.

إعداد : شبكة منهاجي التعليمية

10 / 02 / 2023

حمّل تطبيق منهاجي الجديد

النقاشات

حمّل تطبيق منهاجي الجديد

النقاشات

جميع الحقوق محفوظة © 2025